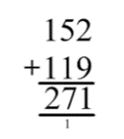10 things I have learned about teaching primary maths to support a pupil with vision impairment

Teachers constantly inspire me. I love watching their development as they learn more about the curriculum they are teaching and as they develop their classroom practice. Great teachers are always growing, reflecting, and adapting what they do to best meet pupil needs.
This is never more evident than when teachers meet a new pupil who has needs that may be different from those that they have come across before. For me, this is when we learn most as teachers and add most to our “teaching toolbox” but it is also when we can feel most vulnerable. I am absolutely delighted therefore, that one inspirational teacher, Sam Hodges, has agreed to share her journey over the last academic year….
When I was told that my new mixed-age Year 3/4 class would include a child who is registered blind, I had many questions going through my head wondering how I would have to change my teaching to ensure her needs were met. My biggest question was around maths.
As a school, we follow the HFL EDucation ESSENTIALmaths mixed-age planning and if you are a user of the planning, I am sure you will be aware of how visual the teaching is, especially in the younger years.
Where would I start?
What would I need to do differently?
In this blog, I will share my teaching journey this year; what I have learnt, how I have developed my practice and how this has supported Olivia to make huge progress in her mathematical learning. Olivia is not the child’s real name but is used for the purpose of maintaining anonymity.
Finding the right starting point
As Olivia was new to school and had limited prior maths teaching, it was essential to establish the right starting point. Based on my knowledge of the maths progression that we follow, I carried out my own assessments with Olivia and found that the best place to start would be building from foundations developed in the EYFS curriculum (Reception ESSENTIALmaths): focusing on building understanding of the number system and the value of numbers.
Using expert advice to build personalised provision
Advice from the HCC Visual Impairment Team identified that repetition is key to ensure learning is secured and that a child who has a visual impairment needs more repetition to secure their learning as they do not have visual aids to refer to around the classroom.
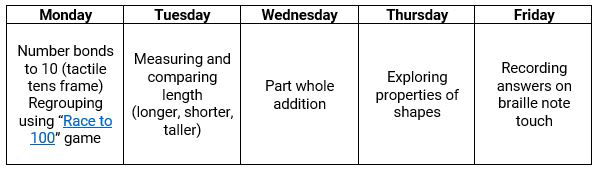
However, I knew that if I had to teach the same maths lesson every day each week, I would get bored of teaching it (let alone being a child doing the same thing each day) so we developed a rolling timetable for Olivia’s maths lesson. We created a carousel of activities that we would repeat each week. An example is shown below.
Every half term, four of these activities changed. However, we felt it was necessary to keep the number and place value activities constant to secure key learning as her understanding continued to grow in this area.
Recognising and adapting to overcome challenges
There were several challenges in supporting Olivia’s understanding of the number system that we came across.
Firstly, where most of us would use our fingers or objects to learn how to count, we would see the amount is increasing. However, for someone who is visually impaired, they do not have the same recognition. We tried many ways to support counting and found that concrete resources were the most beneficial. However, every time Olivia placed one down, she would not find it again on the table so rather than acknowledging she had counted to ten, she may still be at 1 or 2 as she could not feel the rest of the objects that were on the table.
To tackle this, we introduced small, lipped bowls, which reduced the area she was working in so every time she counted one, she placed one block into the bowl. This way she had a reduced space to scan, and she could feel the correct amount as well as scan to make sure she had not missed any.

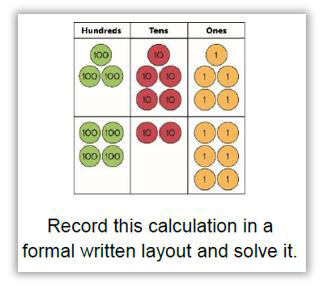
The next challenge we faced was addition.
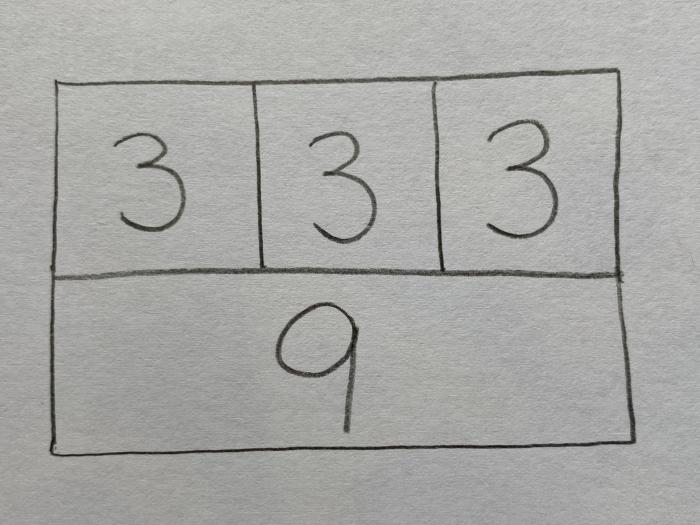
The ESSENTIALmaths planning has a strong focus on the part whole model and regrouping when you get to a multiple of ten. We carefully built learning following the progression: firstly, building security in adding numbers within 10 which could be done using the lipped bowls and cubes. We continued using the lipped bowls and cubes for this, as they were by now familiar resources, and we introduced the concept of two parts making the whole and carefully built from “counting all” towards “counting on”.
To do this, we placed the starting number in one bowl and then asked Olivia to add the second number to it. For example, she would have 5 in the bowl to begin with and would be asked to add 3 more. She was very confident at counting the 5 beforehand to ensure she had the correct amount in the bowl to begin with and was also confident in collecting the 3 cubes she needed to add on.
However, we found that when she added the second part to the bowl and was counting the whole, she was counting some of the cubes twice.
So, at this point, we introduced a second lipped bowl that she transferred the two parts into when adding them together to find the whole. This gave real clarity that the two parts were combining to give the whole and reduced errors.
Building towards independence
One question that we kept asking was, “Have you scanned your bowl?” We were finding that Olivia was telling us all the counters had been moved and she was finished but we would find one counter hiding in the original bowl, so she had to be continuously reminded to scan the bowl to ensure it was completely empty.
This became a question that Olivia is beginning to ask herself and developed meta-cognition towards becoming independent. Through using this method of the bowls, it did not take long until she was secure with her numbers up to 10 and adding within 10.
Building efficiency though connecting understanding
The next step in the progression was to develop understanding of addition beyond 10 and to connect this to place value understanding of the unit of 10. This required a shift of resources, using base ten equipment and regrouping when we got to ten. We knew that we had to build on from what was secure and that plenty of repetition would be needed so we used a game to support this – Race to 100.
The game is modelled on the HFL Education: ESSENTIALmaths YouTube channel and is one that I had already used successfully with other children as a high value intervention as well as within the teaching progression for place value and mental calculations.
I knew that the gaming element supported motivation, frequent practice and focused teaching but also knew that some adaptations would need to be made.
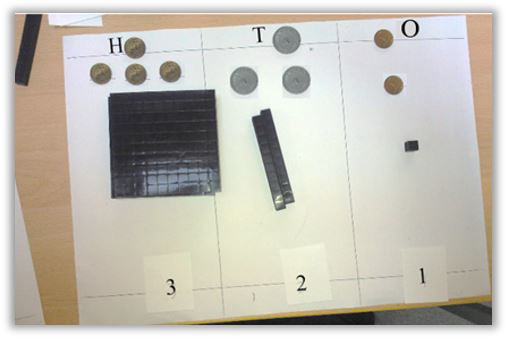
We created a board with brailed labels of ‘ones’ and ‘tens’ and a thin strip down the middle in order to separate the two groups by touch. This provided a working space and supported the development of place value understanding of ones and tens.

Keeping focus on the learning
When we started playing the game, we used an adapted raised dice to indicate the number of ones to be collected. Each time the dice was rolled, Olivia was able to collect the ones and add these to the part that was already known. This was familiar.

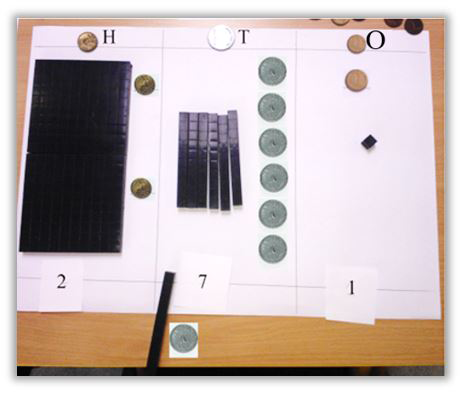
Importantly, I stopped her when she got to 10. At this point, I carefully explained that we could regroup 10 ones for a tens stick. I guided her to feel the tens stick so she felt the 10 dents within the stick and understood that you could place 10 ones on top and they would be the same length as a tens stick. We repeated this a few times and every time we got to 10, we regrouped and discussed what was happening.
Building learning in small steps
Once Olivia was comfortable with regrouping 10 ones for a ten, we moved on to bridging over 10. This became quite confusing, and it took a while for her to grasp the concept. We brought out the lipped bowls and rolled the dice; every time she went above 10, we stopped and spoke about the regrouping.
Although she understood that 10 ones could be regrouped for a tens stick, it took a while for her to understand that she could still have ones left over after regrouping as well. The way we got over this was by removing the ten ones that we regrouped for a ten stick and getting her to scan to feel how many ones were left in the bowl.
Once she had counted how many were left, I had planned for us to then count on from 10. This was not the case though. Instead of Olivia remembering the tens stick had a value of ten, she counted it as 1. It took careful explanation, alongside feeling the resources to check and repetition to move the learning forward.
We repeated the process several times where we bridged over 10 and it took a few sessions for her to remember that the tens stick has the value of ten rather than the value of 1 so she needed to count on from ten. When we then got to 20, we took our time to ensure that she understood that two tens sticks carried the value of 20 rather than 2. However, after this concept was secure, moving on came a lot quicker for her as we worked up the number system.
Building efficiency though developing fact recall
To reduce reliance on counting and to build efficiency, we knew that it was important to support development of fact recall and particularly number bonds to 10. To teach this, we used a tactile tens frame. We started off by allowing Olivia time to feel and explore the tens frame so she understood that there were 10 spaces on it.

We repeatedly used magnetic counters on the tens frame and would place a few on and ask Olivia how many more we would need to count to ten. For this, she had to scan to feel the empty spaces. At the start, a lot of guidance was needed for her to scan systematically across the tens frame as she was counting the empty spaces twice or missing a few.
To challenge her, we then began to place the counters on the tens frame in a random pattern so she would have to feel for the magnets and then feel for the spaces.
We did have to make sure the magnets were strong as Olivia can be very heavy handed and at times ended up moving the magnets as she scanned around. Again, we repeated this weekly for roughly a term and a half and she is now secure on the recall of her number bonds to ten.
Using key questions
One thing I have noticed that has had to change in my teaching is the language I use for questioning. We regularly ask Olivia, “Are you sure?” when she is giving an answer as a prompt to remind her to look back and see if she has worked it out correctly. Unlike most of the class who can see their answer in front of them when using concrete resources, Olivia can’t, so needed to be taught to regularly go back and check she had counted everything.
Adapting provision based on observation to best meet pupil needs
Through observation and working closely with Olivia’s 1:1 support and the Visual Impairment team, we came to a decision to slow the lessons down and reduce the time of her lessons to 40 minutes. This ensured we focused on the scanning aspects so that was not rushed, as well as identifying how tiring maths lessons were for her and acknowledging that she could not keep her concentration for much longer due to the amount going on within the lesson.
We have also made a conscious decision to regularly introduce new language throughout lessons. After a few times of the word or phrase being spoken by the adult in the lesson, Olivia can pick it up and begin to use it, although this is still a ‘work in progress’ as she does need reminding quite a bit to do this.
Reflecting on what has been learnt and successes
This year has been an excellent experience and a fantastic learning opportunity for me to teach someone who is visually impaired. It has taught me many things that I will continue to use to support my teaching. The learning journey Olivia has been on throughout the year has been lovely to watch and it has been amazing to see her confidence in maths grow. As well as this year teaching me a lot of new things about teaching, it has also helped the whole class; their use of language while explaining has come along well as they know they need to explain their answer in enough detail so that the child who is visually impaired understands their answer.
I am excited to see where the next stage of Olivia’s learning takes us as a team. I look forward to continuing to support her understanding of maths as well as expanding my understanding of strategies of teaching for someone who is visually impaired.

Providing high quality practical experiences that build on children’s interests linked to the world around them can provide enriching learning opportunities that will support learning across all areas of the EYFS curriculum as well as improving children’s final outcomes in the Early Years Foundation Stage Profile (EYFSP).












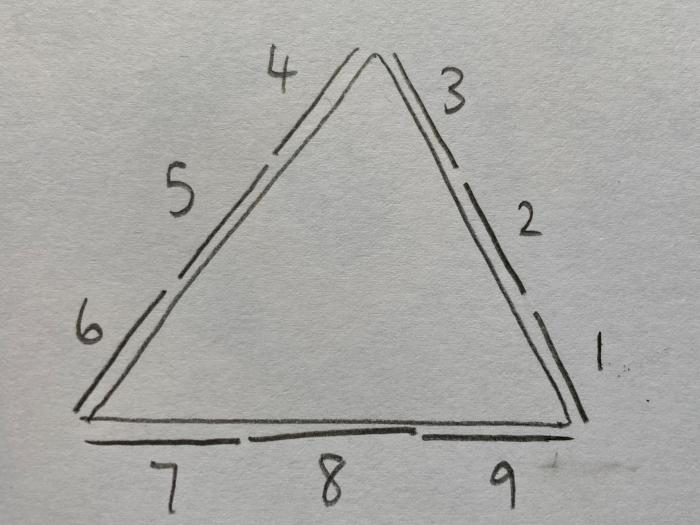




-exploring-the-differences-2.JPG)
-exploring-the-differences-3.JPG)
-exploring-the-differences-4.JPG)
-exploring-the-differences-5.JPG)
-exploring-the-differences-6.JPG)
-exploring-the-differences-7.JPG)
-exploring-the-differences-8.JPG)
-exploring-the-differences-9.JPG)
-exploring-the-differences-10.JPG)
-exploring-the-differences-11.JPG)