The simple bead string is one of the most common classroom resources I see in primary maths classrooms.
The basics
Bead strings commonly come in two different types.


100 beads with bead colour alternating in units of ten
20 beads with bead colour alternating in units of five (most commonly used in Year 1)
Top tip: The quality of the string is important.
Some bead strings are designed so that the beads will stay in place when held up. Even if the bead string is held vertically, the beads can be released by pulling the string tighter. This makes them much easier for both the teacher and the pupils to use, as the beads don't slide around.
Uses for a 0-100 bead string are wide and varied. This blog focuses on how to introduce it and familiarise pupils with it so they can get the most out of it.
Health and safety – how to introduce the bead string safely
It is important to set firm ground rules when introducing the 0-100 bead string to pupils. In my time, I have seen a few pupils behaving unsafely with them in the classroom.
Tips for introducing the 0-100 bead string
- Only introduce one new manipulative at a time
- Be clear with pupils that they are not to touch the bead strings unless they have been instructed to. If they find it hard not to fiddle, and the fiddling is distracting either their own or others' learning, ask the pupils to place the bead strings on the floor and not to pick them up until instructed to.
- Be clear about what they are using the bead string for. It is to help them with their maths, not for skipping, swinging, or tying up their friends!
- Allow them time to explore and 'play' with the bead string, then lead them into guided discovery.
Top tip: Depending on which end of the bead string you start with, the first unit of ten might be white, or it might be red. This is a potential problem for struggling pupils, as the bead string the teacher is modelling with can look very different from the bead string they are holding.
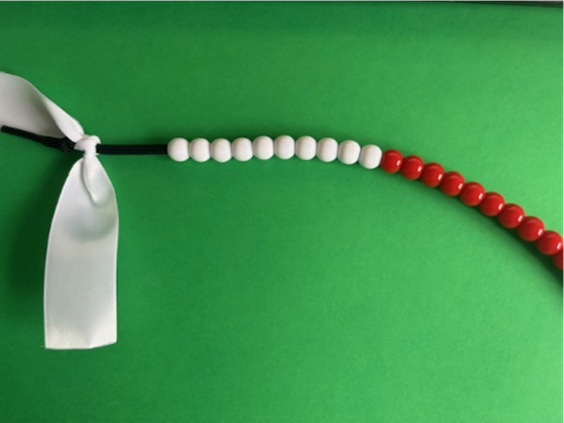
Fortunately, this can be overcome by tying a piece of ribbon to the end you want them to hold in their left hand and explicitly showing them which end to call zero to help them match the teacher's modelling.
Guided discovery
Help pupils get more familiar with bead strings by asking questions that support them to notice and make connections.
- Why are the beads different colours?
- Is there a pattern to the colour of the beads?
- How many beads are there on the bead string?
- How might this help with addition and subtraction?
- When else might the bead string help?
Regular exercises with the bead string
Problem: pupils count the beads one at a time to find a number
Pupils need to develop quick and efficient ways of finding numbers on the bead string. Fortunately, the unit pattern can help, but pupils need to be explicitly taught this and have regular practice.
Not only do these exercises help them find numbers quickly on the bead string, but they also impact their number magnitude development (including in rounding and estimating) and their mental calculation (moving from ‘counting all’ to ‘counting on’).
Solution: familiarisation with tens and ones
- Count up and down the bead string in tens
- Show me in one movement:
… 10 (encourage showing the first unit of ten in one movement)
… 20 (encourage showing the first two units of ten in one movement)
… 100 (showing the whole bead string)
…0 (showing the start of the bead string without any beads slid across)
… 90 (encourage moving back one unit of ten from the 100)
… 80 (encourage moving back two units of ten from the 100)
Once the pupils are confident with the unit blocks above, they can be extended to showing the other decade numbers (30, 40, 50, 60, 70). Pupils may not be able to find these immediately but should count the units of ten.
Teacher: Show me 40.
Pupil: 10, 20, 30, 40 (counting the units of ten) – here’s 40.
- Show me in as few steps as possible:
… 32 (encourage sliding three units of ten (separately or as a set) and then subitising the ones)
Pupil: one ten, two tens, three tens – that’s 30… and two more – that’s 32.
… 47
… 63
Once pupils are confident with the above exercises, we can move on to improving their efficiency and deepening understanding of numbers.
Examples of strategies pupils might use
Spend time discussing different strategies the pupils use in the classroom, encouraging them to think about which ones are more efficient, and then letting them practise.
Moving towards efficiency
Show me two ways to find (select numbers that are near to decade numbers):
… 9
Strategy 1: one, two, three, four, five, six, seven, eight, nine
Strategy 2: One ten… and one fewer – nine
… 18
Strategy 1: one ten… and one, two, three, four, five, six, seven, eight
Strategy 2: two tens (20)… and two fewer – 18
… 97
Strategy 1: one ten, two tens, three tens, four tens, five tens, six tens, seven tens, eight tens, nine tens – that’s 90… and one, two, three, four, five, six, seven
Strategy 2: one hundred… and three fewer – 97
… 72
Strategy 1: one ten, two tens, three tens, four tens, five tens, six tens, seven tens – that’s 70… and one, two
Strategy 2: one ten, two tens, three tens, four tens, five tens, six tens, seven tens, eight tens – that’s 80… and eight fewer – 72
This last example demonstrates to the pupils that it is not always most efficient to go to the next decade number and then adjust down. However, it is still very good mental agility practice for them.
Show me three ways to find 50.
Strategy 1: Count up in tens.
Strategy 2: Count back in tens.
Strategy 3: Fold the bead string in half.
Once pupils are confident with identifying 50, this can increase efficiency further. For the final example:
… 72
Five tens, six tens, seven tens – that’s 70… and two more
Extending the exercises through key stage 2
Regularly rehearsing finding numbers on a bead string is not restricted to Year 2 and Year 3. By renaming the beads' values, these exercises can be extended into other year groups.
Year 3
Rename the bead string 0 to 10.
- What is the value of each unit of colour now? (1)
- What is the value of each bead now? (one tenth, 0.1)
- Count up and down the bead string in tenths.
Examples for Year 3:
Step 1 | Step 2 |
Show me in one movement | Show me in as few steps as possible |
2, 3, 9 | 4, 7, 8 |
Step 3 | Step 4 |
Show me in as few steps as possible | Show me two different ways to find |
3.1, 4.7, 6.3 | 9, 1.8, 9.7, 7.2 |
Year 4
Rename the bead string 0 to 1.
- What is the value of each unit of colour now? (one tenth, 0.1)
- What is the value of each bead now? (one hundredth, 0.01)
- Count up and down the bead string in hundredths.
Examples for Year 4:
Step 1 | Step 2 |
Show me in one movement | Show me in as few steps as possible |
0.2, 0.3, 0.9 | 0.4, 0.7, 0.8 |
| Step 3 | Step 4 |
| Show me in as few steps as possible | Show me two different ways to find |
| 0.31, 0.47, 0.63 | 0.9, 0.18, 0.97, 0.72 |
Year 5 and Year 6
Rename the bead string 0 to 100%.
- What is the value of each unit of colour now? (ten percent, 10%)
- What is the value of each bead now? (one percent, 1%)
- Count up and down the bead string in percentages.
Examples for Year 5 and Year 6:
Step 1 | Step 2 |
Show me in one movement | Show me in as few steps as possible |
20%, 30%, 90% | 40%, 70%, 80% |
| Step 3 | Step 4 |
| Show me in as few steps as possible | Show me two different ways to find |
| 31%, 47%, 63% | 90%, 18%, 97%, 72% |
Are you looking to enhance the way your primary school pupils learn and engage with mathematics? Our dedicated primary maths teaching and learning advisory team is here for you.