
How can Cuisenaire Rods help us to develop maths fluency?
They can of course be used to add and subtract numbers with children using counting strategies and number tracks. However, Cuisenaire Rods are a great resource to help expose the structure behind calculation strategies and support children to move on from being reliant on counting.
In this blog, we will explore how Cuisenaire Rods can support:
- understanding of reordering and adding complements to key benchmarks;
- using compensation when adding and subtracting;
- exploring the strategy of equal difference.
One top tip before we begin…
If children are unfamiliar with the value of each rod, lining them up with a ruler can be really useful! The videos embedded in this blog model this.
Reordering and complements to benchmarks
What is the easiest way to find the total of 3 + 5 + 7?
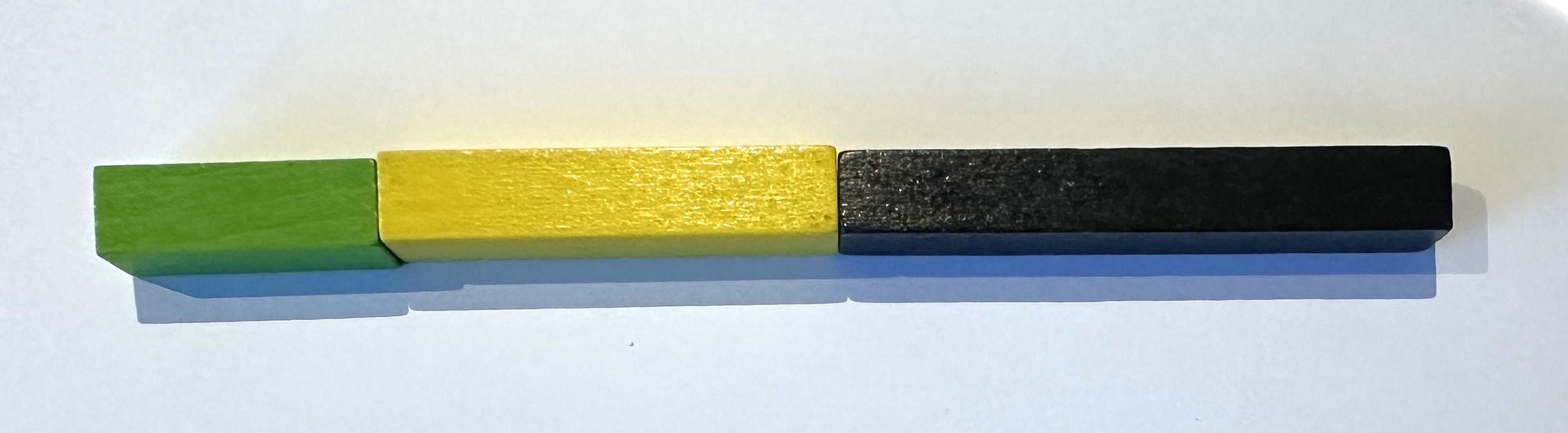
Choosing an appropriate calculation strategy is all about making the maths easier for ourselves! In this case, using what we know about complements to 10 (a helpful benchmark) may be a useful strategy. The following video shows how we might model and expose this strategy.
Once the calculation strategy has been exposed and understood using 1-digit numbers, this can then be applied to calculations using larger numbers
So, in Year 2 (and subsequently), we could use this strategy to explore complements to 100. The green rod was worth 3 ones but let’s say it is now worth 3 tens or 30. The yellow rod is worth 5 tens and the black rod is worth 7 tens.

30 + 50 + 70 could be reordered so that the complement to 100 is found (30 and 70) before adding the 50. Children should be encouraged to reason that: ‘I know that 7 tens and 3 tens is 10 tens or 100, and then I will add the other 5 tens’.
Each time we model, it would be worth reminding the children that we are using a strategy to make the maths easier because we are using our known facts.
In Year 4 (and subsequently), exploration can progress to fractions and decimals.
Finding complements and reordering could be a useful strategy for Year 4 pupils learning to add fractions with the same denominators.
In Year 5, we can apply this to adding decimal amounts. In these next two videos, rather than representing a one, the white Cuisenaire Rod is used to represent tenths and then fifths.
Compensation (9 is near 10)
What is compensation?
When we add 9, we may think to ourselves, ‘9 is close to 10, so I can add 10 and then subtract 1’. We are changing the amount we have added (10 instead of 9) and so we need to compensate after adding 10 by subtracting 1.
‘I have added 10 which is too many. 9 is 1 less than 10 so I need to subtract 1.’
How can we use Cuisenaire Rods to expose this strategy?
What about if we were to subtract?
Let’s take the calculation 15 – 9. Many children may be able to recall this as a known fact. However, this is a useful calculation to start with when wanting to expose the structure behind the strategy. The following images and language show how this could be modelled using Cuisenaire Rods:

‘9 is 1 less than 10 so subtracting 9 is very close to subtracting 10. I can subtract 10 from 15’.
When modelling this, we could use the orange rod to represent the 10. Then replace this with the blue and white rods to show that 10 is also represented by 9 and 1.
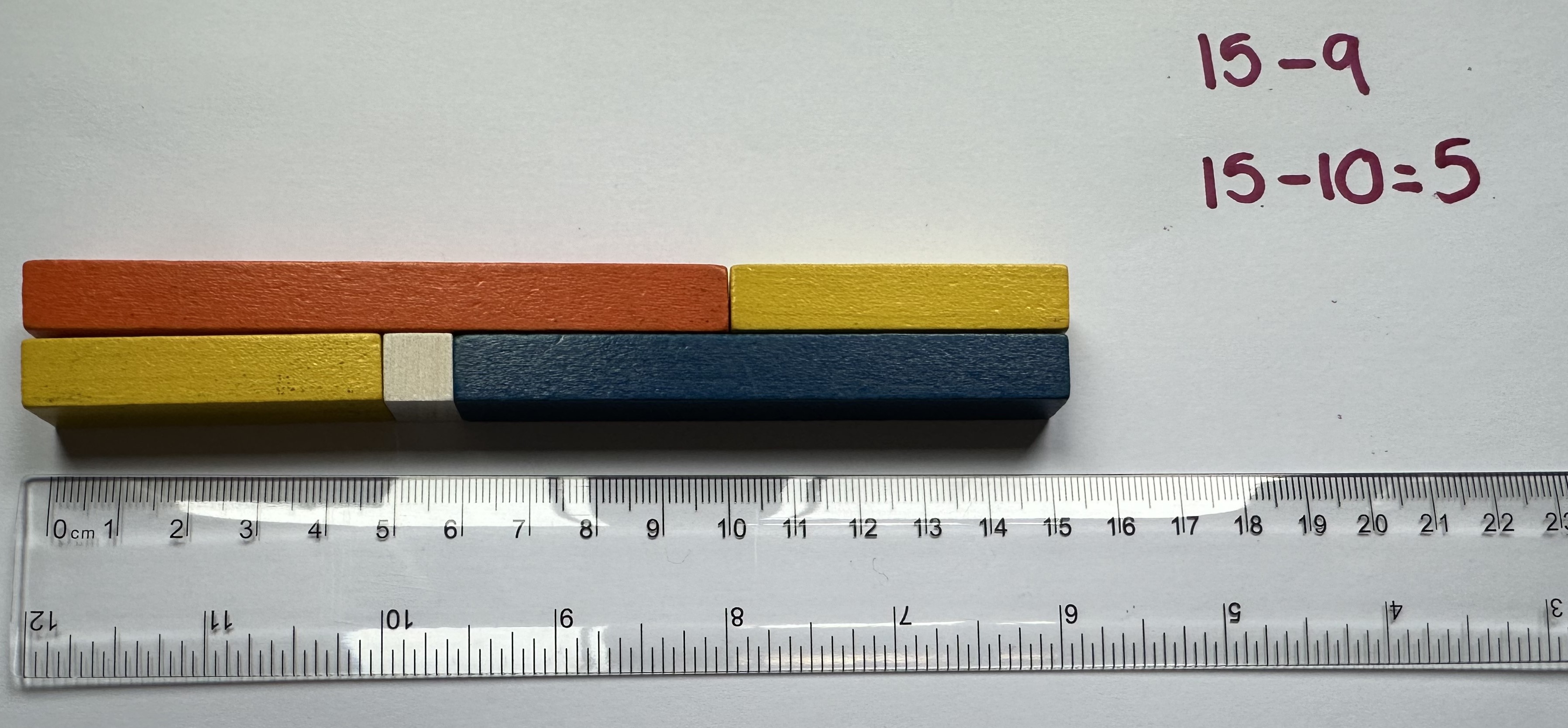
‘15 subtract 10 is 5. I can use my place value knowledge for this. 1 ten and 5 ones subtract 1 ten, leaves 5 ones’.
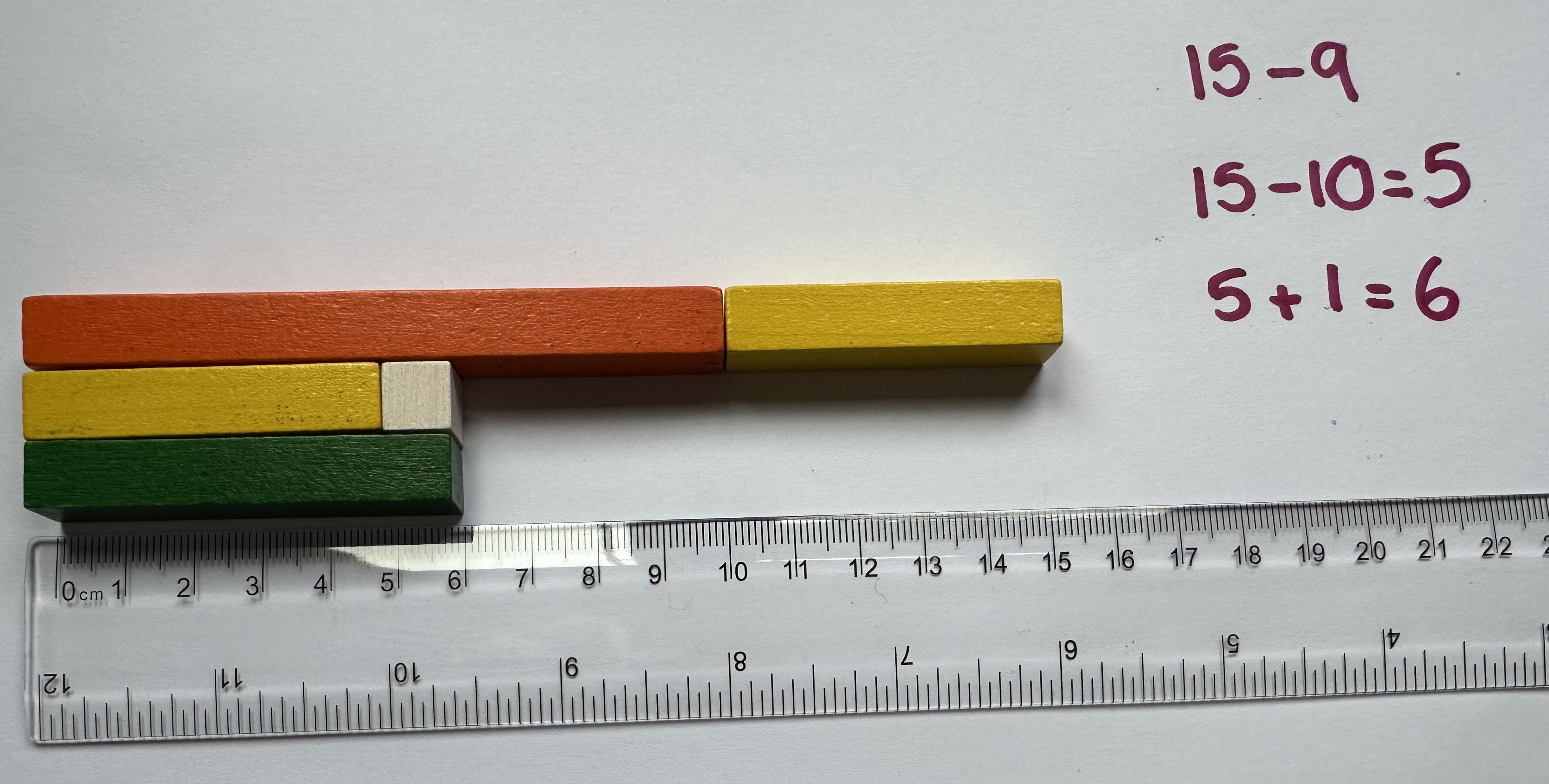
‘I have subtracted 10 which is 1 more than 9 so I need to add 1 back on. 5 + 1 = 6’.
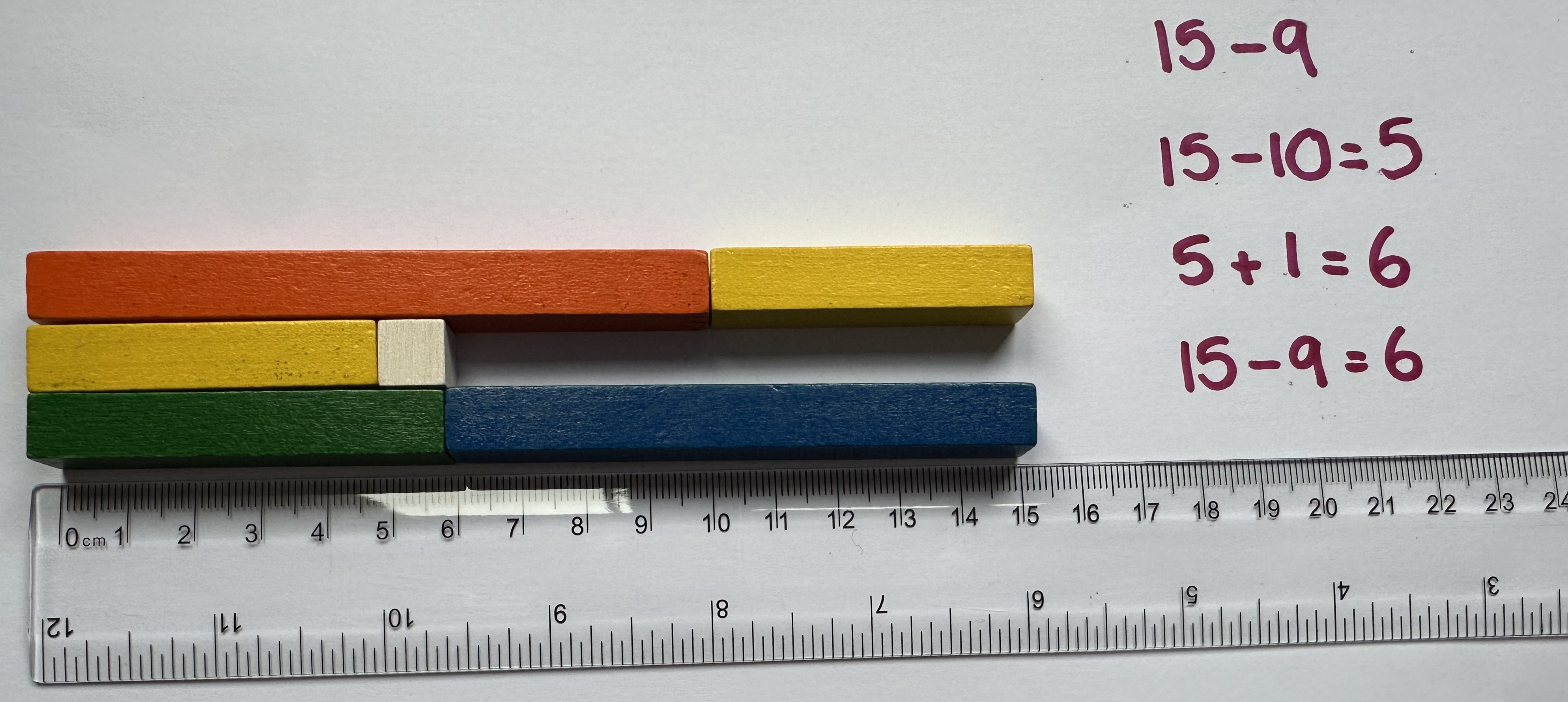
’15 – 9 is equal to 15 – 10 + 1.
15 – 9 equals 6’.
Once the calculation strategy is exposed and understood, children can explore more complex calculations. For example, using compensation to calculate 3562 – 198 could look and sound like this:
‘Subtracting 198 is like subtracting 200 and then adding 2 back on.
3562 – 200 is 3362. 3362 + 2 is 3364. Therefore, 3562 – 198 = 3364’
Exploring equal difference
If we needed to subtract 9 from 17, perhaps it would be easier to represent the calculation as 18 – 10.
How does this work?
To make the calculation easier, we have added 1 to both numbers and this maintains the same difference. The difference between 17 and 9 is equal to the difference between 18 and 10. This is called ‘equal difference’ and is an alternative method to compensation.
In the compensation strategy, we adjust after we have performed the subtraction. Whereas in equal difference, we adjust first, and then perform the subtraction.
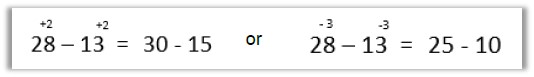
It is important children understand that if we add or subtract the same amount from both numbers (the minuend and the subtrahend) then the difference remains the same.
In the example here, by adding 2 to both 28 and 13 we get 30 – 15 which the children may be able to use known number facts to solve. Alternatively, we could subtract 3 from both numbers to get 25 – 10.
This is about finding more manageable ways of solving the calculation and that choice may be different for different children.
The following video shows how equal difference could be explored using Cuisenaire Rods before this knowledge is then applied to calculations.
Now that we have explored these strategies, are there other strategies that you could use Cuisenaire Rods to explore? I wonder what near doubles would look like with Cuisenaire Rods? Or ‘Think 10’?
Find out more about the HFL Education Curriculum Impact Packages (pdf)
To keep up to date: Join our Primary Subject Leaders’ mailing list
To subscribe to our blogs: Get our blogs straight to your inbox



