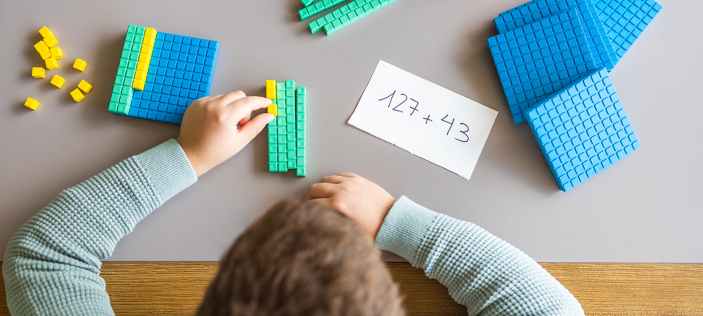
In my previous blog, KS1 mathematical recording is not just for Ofsted I considered the question that I am often asked by teachers when reflecting on their maths teaching, “What should it look like in their books?” This led to reflection as to the purpose of recording in maths. I concluded that recording is essential, although how this is achieved depends on the purpose of the mathematical recording - whether it aims to make connections between models, practise a new skill, record the journey through a problem, develop precision in reasoning, focus on reflection and evaluating strategies...
In this blog, I have tried to capture some ideas which might support teachers (KS1 especially) to develop manageable and meaningful mathematical recording. What follows are some practical suggestions and examples of what is achievable in KS1. All examples show work produced by Year 1 children and have been provided by some of the amazing practitioners that we have the pleasure of working with. They show how recording can be manageable and meaningful and support pupils to deepen their understanding of maths; they show just how important recording in maths can be.
Encourage pupils to record alongside concrete models
Through supporting pupils to move from concrete to abstract, through pictorial representations, mathematical understanding is deepened. In this example from Ashtree Primary School in Stevenage, I was delighted to watch as pupils chose their level of challenge and then busily built their part whole models, recording them independently.
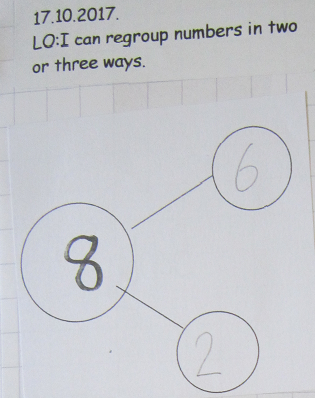
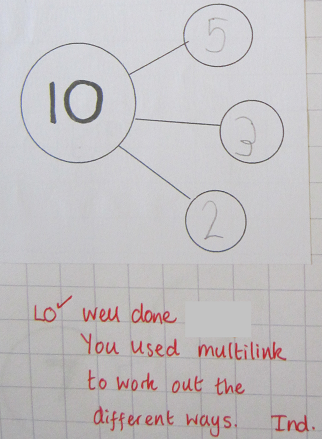
Encourage pupils to record pictorially and abstractly together
In addition to supporting transfer between models to build depth of understanding, recoding pictorial representations alongside abstract ones, ensures that those pupils who find recording difficult can still prove their mathematical understanding and provides an opportunity to practise the elements of recording the pupil is finding hard.
For example, the pictorial representation of 31 below, produced by a Year 1 child from St. Paul’s Catholic Primary School in Cheshunt, leaves no room for doubt over the intention of the pupil and allows the teacher to identify that further practise in writing the numeral 3 is required and provided.

In the example below, from St. Alban & St. Stephen Catholic Infants, pupils have used abstract representations to show understanding of the connection between the part whole model and addition and subtraction.
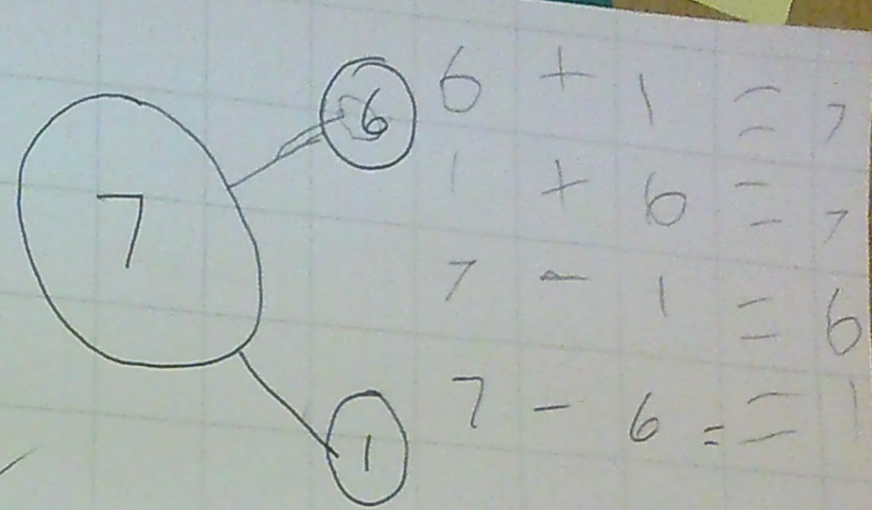
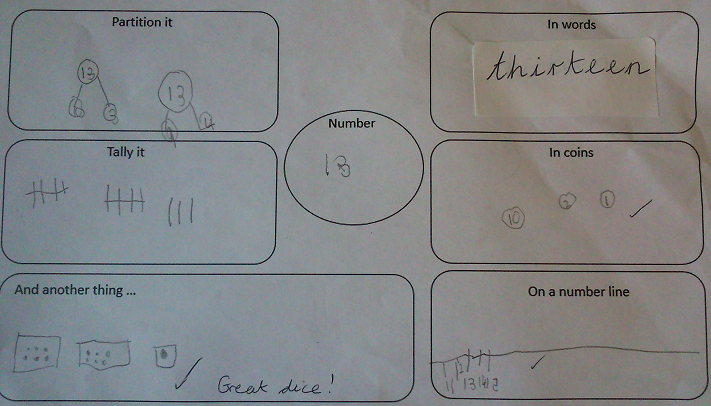
Develop depth of understanding through encouraging multiple representations
In the example below, from Boxmoor Primary School in Hemel Hempstead, the pupil is recording their understanding in many different ways including using their own representation and each different representation is exposing something different about their understanding.

Similarly, from the same school, the representations below all show the same thing, but each tells us something different about what the child understands. For example the tens frame model showing understanding of the two parts of the whole, the part whole model emphasizing the understanding that the parts go together to create the whole and the bar model highlighting understanding of the relative sizes of numbers.
Provide pupils with opportunities to complete representations to show understanding
Often activities can be “tweaked” so that the amount of recording needed is limited or to enable pupils to record in different ways, for example by completing representations. This example from Millbrook School in Cheshunt, provides an excellent example of just that and ensures that the focus can be placed on showing mathematical understanding.

Similarly this example, from St. Gregory’s Catholic Primary School in Northampton, has provided the opportunity for the Year 1 pupil to complete the representation to show understanding.

Provide opportunities to annotate representations to focus on reasoning
When the focus of the recording is reasoning, providing representations in questions can enable children to be able to annotate what is already there to show their understanding and so the focus can be placed on explaining how the pupil knows.

Encourage pupils to prove how they know
Proving does not need to involve words. Consider how, in the example below, the pupil has proved that one more than 12 is 13.
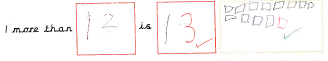
However, Year 1 pupils can reason in writing. In the example below from St Paul’s, the pupil has proved their understanding pictorially and in writing.In addition, it has given the teacher an opportunity to provide the pupil with precision.

Support pupils to develop independence in recording
At St Teresa’s in Borehamwood, pupils have access to blank tens frames and part-whole models which they can use at any time to support their recording; developing both independence and mathematical recording.
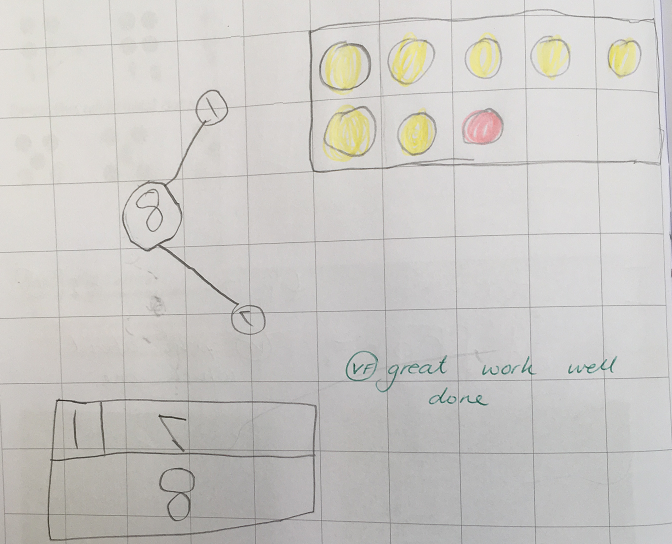
Use questions with limited recording to uncover understanding that is not yet secure
In the question below, pupils are being asked to think deeply about the structure of the problem, but the recording required is limited.
Use recording to challenge
Using the example above, challenge could be added by asking pupils to prove their selection or by considering how else the problem could have been presented. What could the worded problem be? Challenge can often be offered by simply using the command: show me another way.
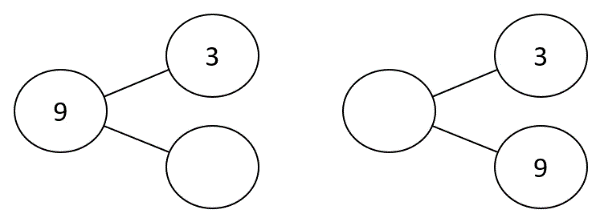
Support pupils to know how the recording could look through modelling
Through models on working walls and providing recording scaffolds, pupils can be shown how the recording might look. Where these are used most effectively, pupils will use them repeatedly from many different starting points and will start to develop their own links too. Having used the same recording frame many times, starting at different points, pupils have the opportunity to reinforce understanding, make new links and develop independence.
Give pupils the mathematical vocabulary
vocabulary precisely is essential in the development of a mathematician. By providing the mathematical vocabulary and using this recorded task, the teacher has made the focus the use of accurate mathematical language.
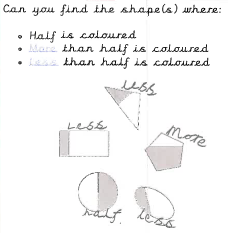
Give pupils the language to communicate mathematically
Pupils need to be supported to talk mathematically before they will be able to record in this way and we know that the quality of this talk is crucial. This is one of the reasons that speaking frames are so integral to the new ESSENTIALmaths resources. The talk has value in itself and so will not always be recorded, but the same speaking frames used to support the talk, can be used as writing frames where appropriate. In addition, talk can be captured where this is purposeful. Some schools achieve this by using post-it-notes or stickers written on and stuck into books or by recording directly into the book.
I am not advocating that this be used all of the time for every child, but it could be a great use of an adult in the room as it helps you assess understanding that you may not have seen yourself and it builds self-esteem for the child. It can also help everyone to focus on the importance of reasoning rather than just the answer. When considering capturing talk you might consider:
- When is this most achievable and beneficial? Carpet time/small-group discussions/during practical activities…
- Who would it be useful to focus on?
- How will talk be captured?
In the examples below, key mathematical noticing and explanations have been captured effectively by other adults in the room.


Other schools use i-pads or other recording devices to capture the rich reasoning in their classes and to offer the children a different audience and medium to share their recording through.
Remember recording DOESN’T have to be all about books…
Pupils tend to like recording in unusual places. Sometime the allure of a post-it or coloured sugar paper can work wonders and can allow for collaboration and recording on the run …
In the example below, pupils worked together to consider the number nine and all the ways that they could record this. Whilst the post-it note came from a quick challenge question from a teacher who said “4 is 4 less than 8. How else could we say that?”
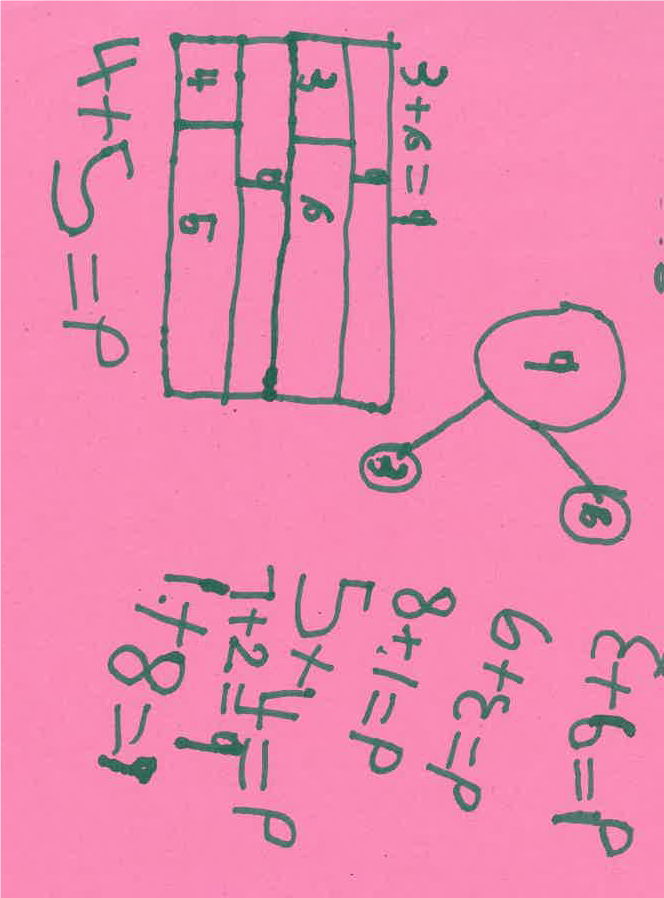

Value recording for the maths
KS1 pupils sometimes identify the best mathematicians as those who record most neatly and this can be a telling sign of what we show that we value in our classrooms. It can be important to make sure that we are show how we value recording which really shows the mathematical understanding.
In summary, I would suggest that we enjoy developing mathematical recording with our pupils, thinking carefully about the purpose that the recording has in each case and ensuring recording provides opportunities for all pupils to access tasks independently, develop their understanding and to demonstrate this. If we focus on the needs of our pupils and developing their mathematical understanding, we won’t be caught in the trap of thinking that we need to record to provide evidence for Ofsted!
Top tips for developing manageable and meaningful maths recording in Year 1
- Encourage pupils to record alongside concrete models
- Encourage pupils to record pictorially and abstractly together
- Develop depth of understanding through encouraging multiple representations
- Provide pupils with opportunities to complete representations to show understanding
- Encourage pupils to prove how they know
- Support pupils to develop independence in recording
- Use questions with limited recording to uncover understanding that is not yet secure
- Use recording to challenge
- Support pupils to know how the recording could look through modelling
- Give pupils the mathematical vocabular
- Give pupils the language to communicate mathematically
- Remember recording DOESN’T have to be all about books…
- Value recording for the maths