
In part 1, we used the bar model to exemplify how to solve questions from reasoning paper 2 from the Year 6 SATs 2024.
You guessed it… in this blog, we will explore questions from the KS2 SATs Reasoning Paper 3.
As Charley mentioned in part 1, a secure understanding of the relationship between parts and the whole is crucial. This learning begins in the Early Years and is built upon throughout Key Stage 1 and Key Stage 2 so please don’t be put off if you don't teach Year 6!
As well as understanding the relationships between part and whole, children also need time to explore these links in context.
Using the concept of ‘start, change, result’ can support children with overreliance on looking out for ‘trigger words’ and ‘underlining important parts’ (remember RUCSAC?!).
We know that when tackling worded scenarios, many errors occur when comprehending the ‘maths’ in the problem and no amount of underlining important parts is going to help.
Instead, ‘start, change, result’ supports children to ascertain the ‘knowns’ and ‘unknowns’ in a problem.
When this is coupled with the use of the bar model, comprehending the ’maths’ in the problem, becomes easier.

In the image above, we can see how we have started with the 8 card and then added a 7 card (this is the change), with the result being that we now have both cards. This could then be linked to the number sentence 8 + 7 = 15 and the bar models below:

The example below shows how ‘start, change, result’ can be used to support more complex structures – in this case, where the start is unknown but the change and the result are knowns. These could then be matched to worded problems.
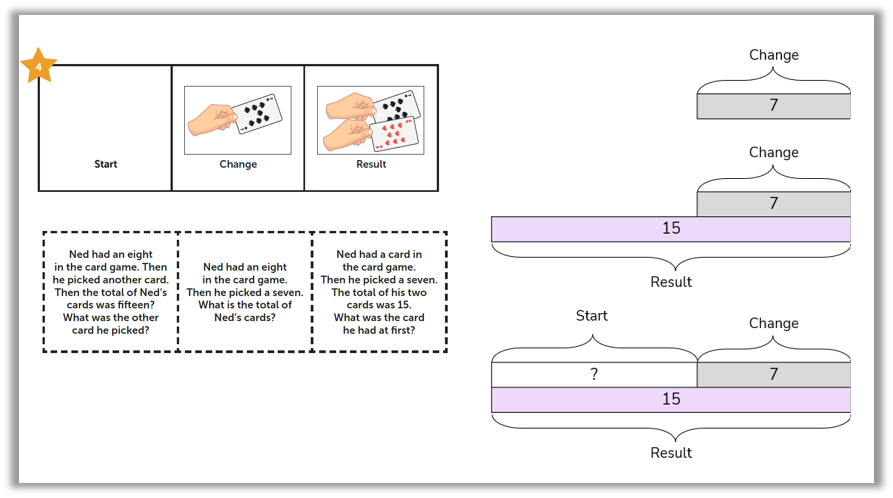
Using the ‘start, change, result’ strategy (which admittedly deserves its own blog!) is a gateway into exploring more complex problems – the sorts of problems that children are likely to come across at the end of Key Stage 2.
As with the first blog that explores paper 2, dialogue and key questions that could be used to support and refine children’s thinking is outlined for each question below from paper 3.
Once again, the language of part and whole is key.
As you read on, you may also like to consider how ‘start, change, result’ could be incorporated, as well as ‘knowns’ and ‘unknowns’.
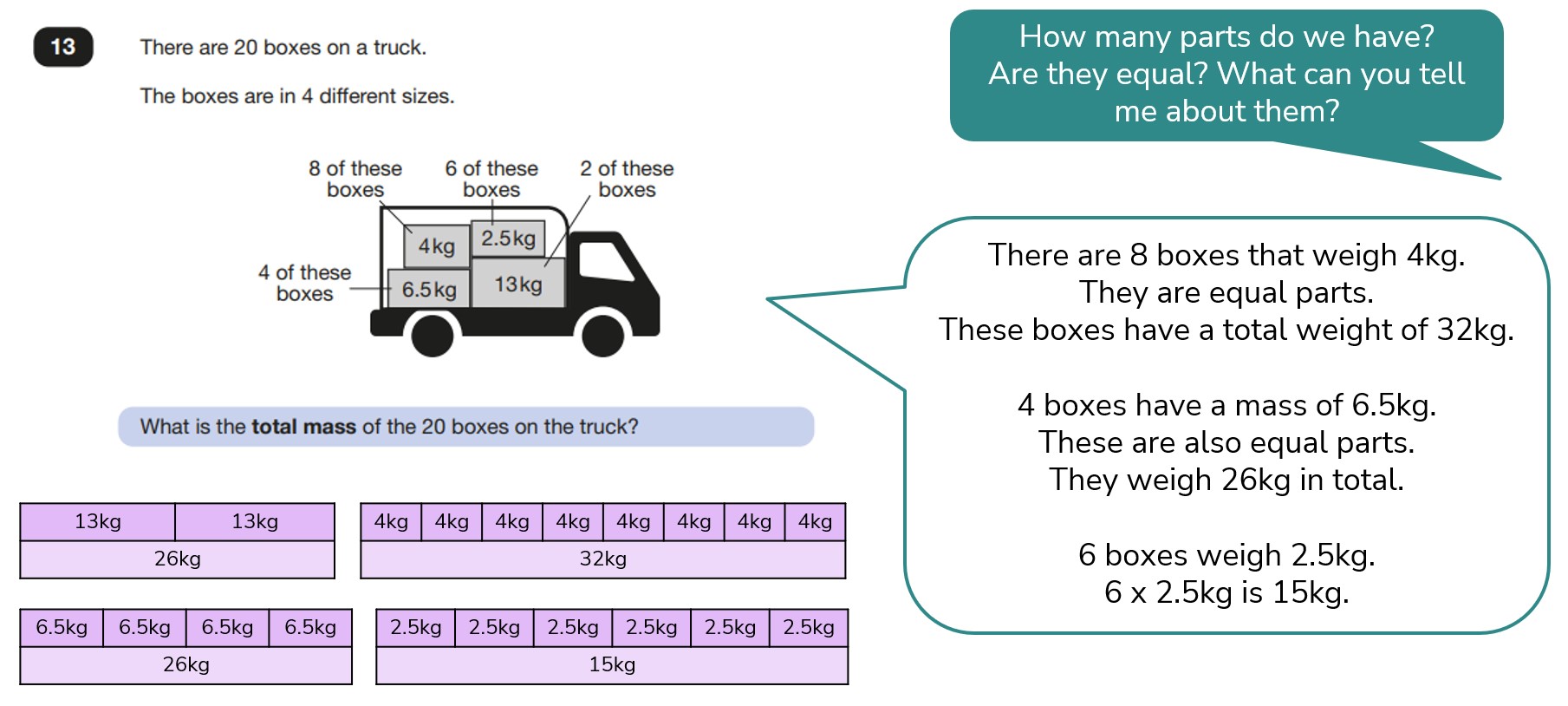
Question 13 – bar modelling to expose the need for multiplication and addition
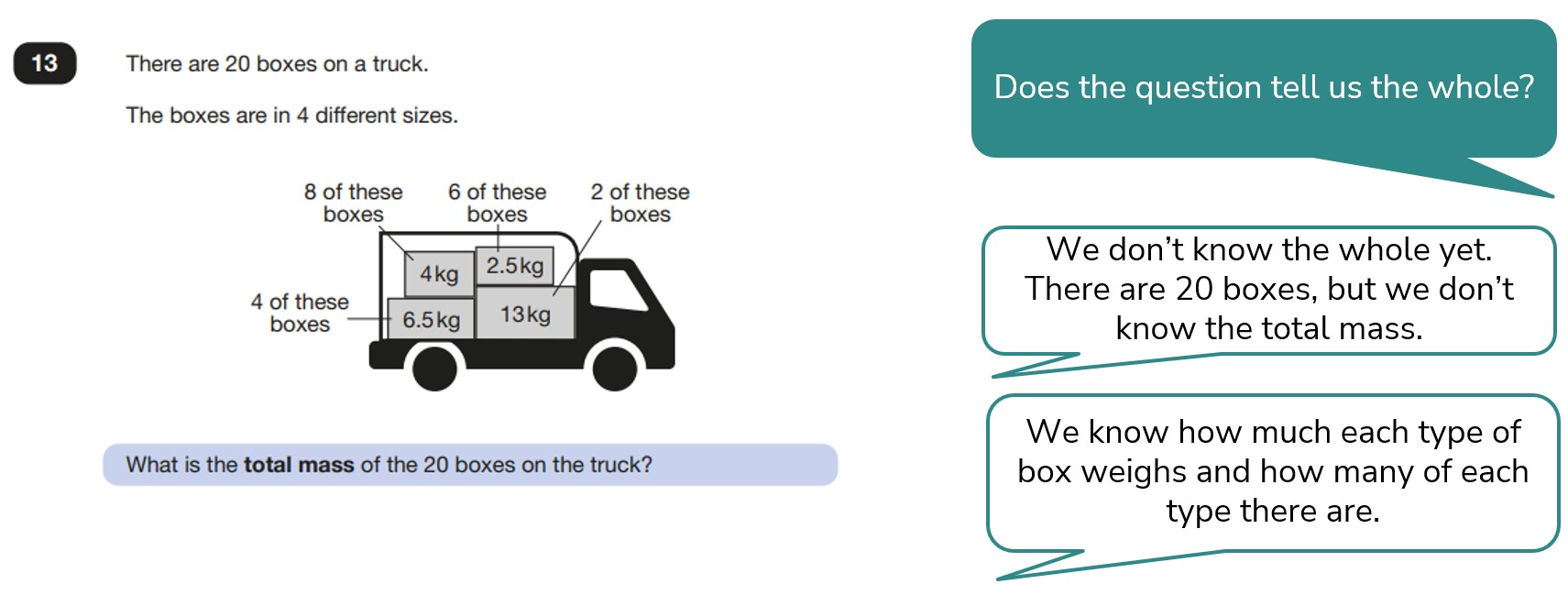
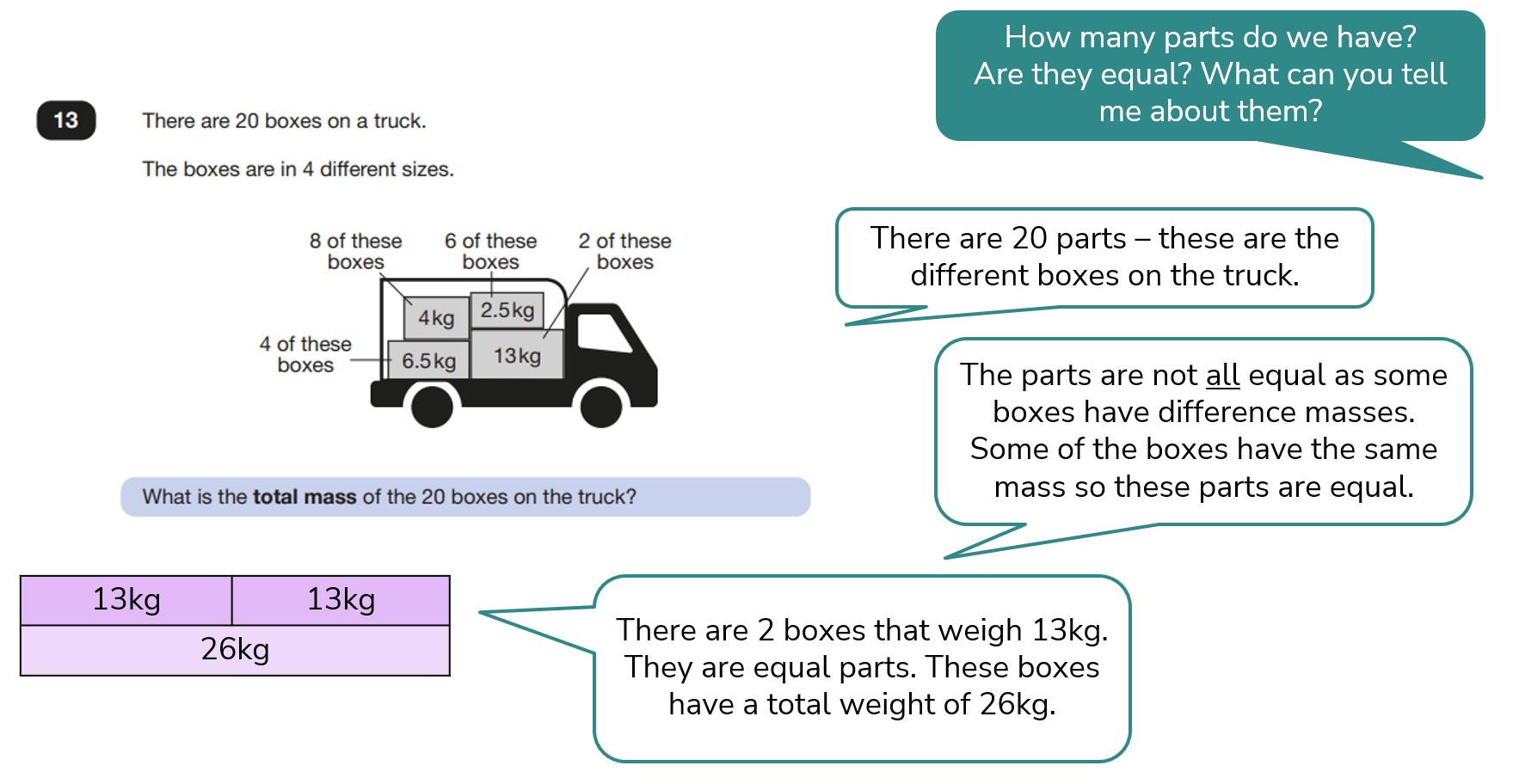
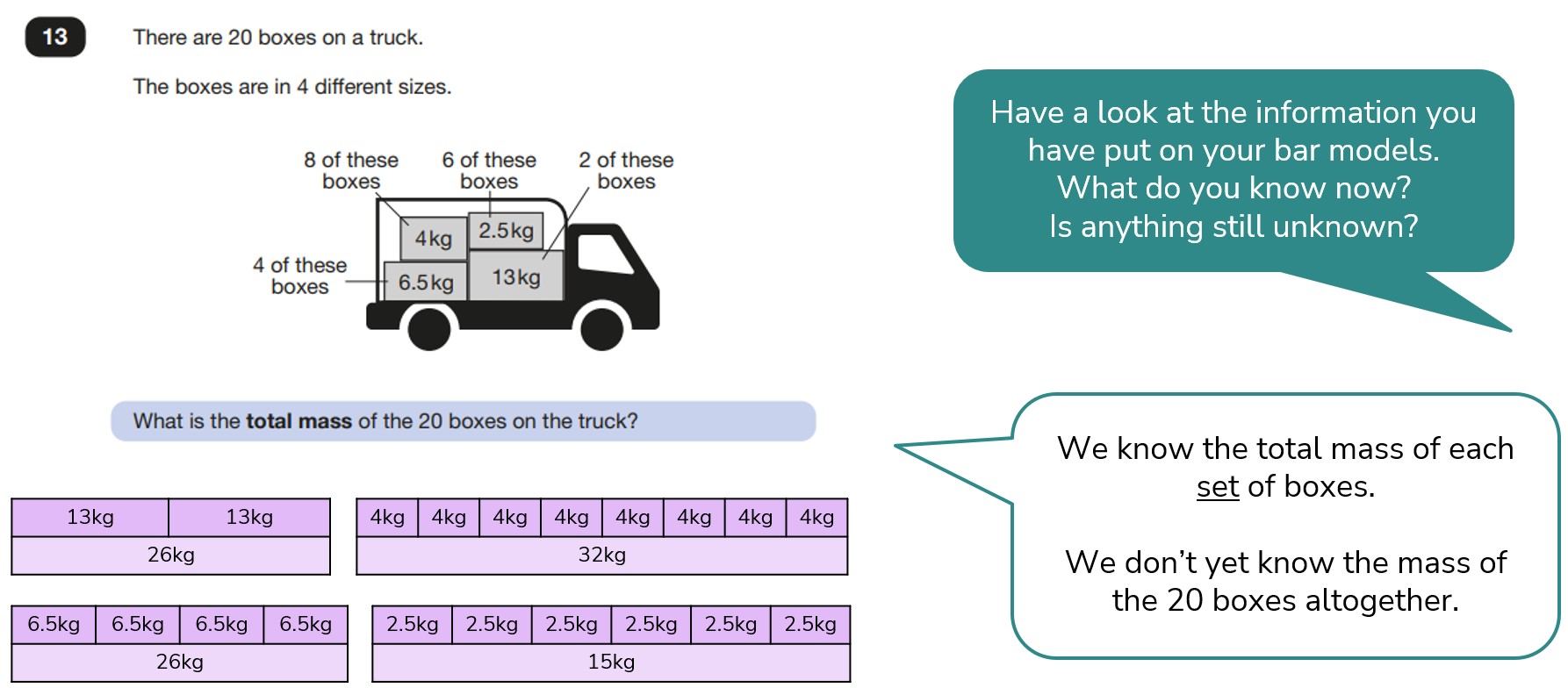
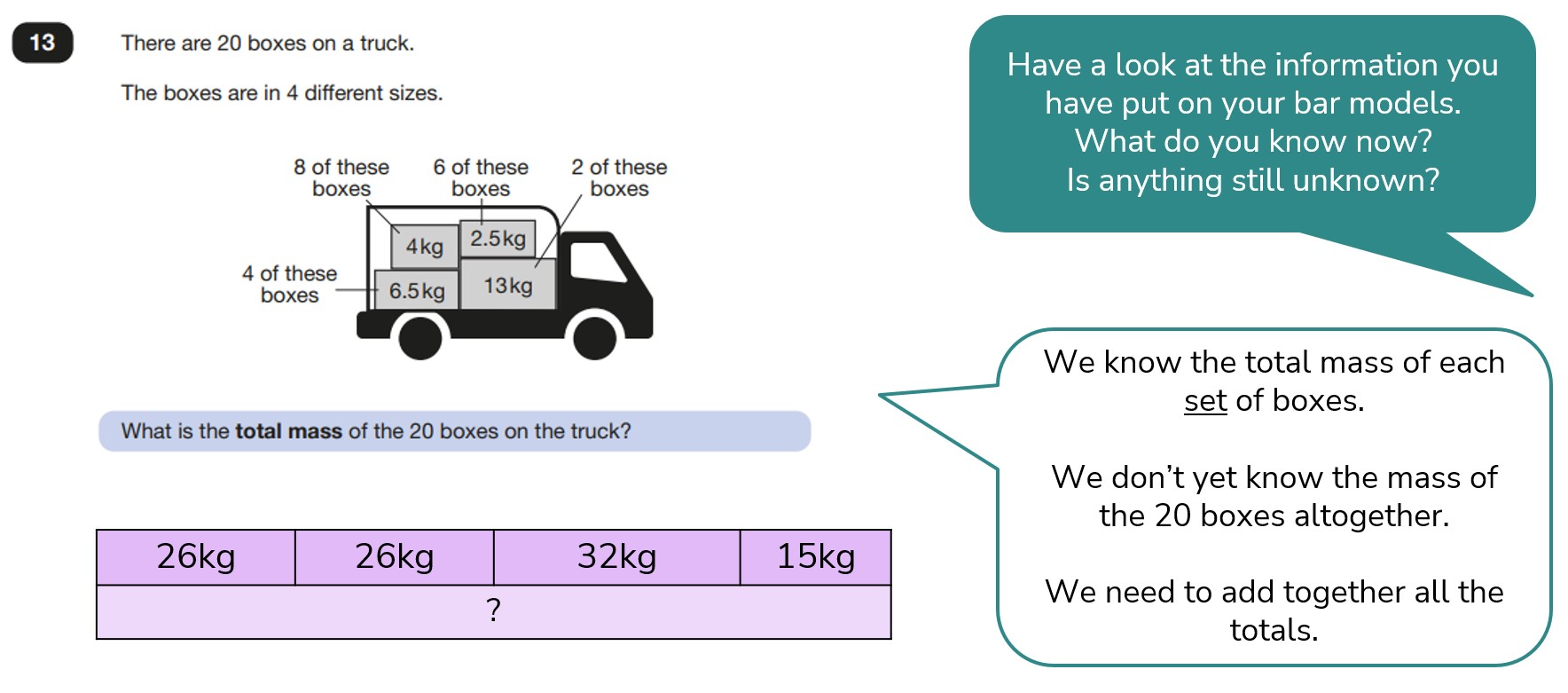
Solution
The total mass of the boxes is 99kg.
In this question, the bar model supports understanding that several multiplication calculations need to take place before adding the products to find the total mass.
15 – bar modelling to expose the need for all four operations within the context of percentages


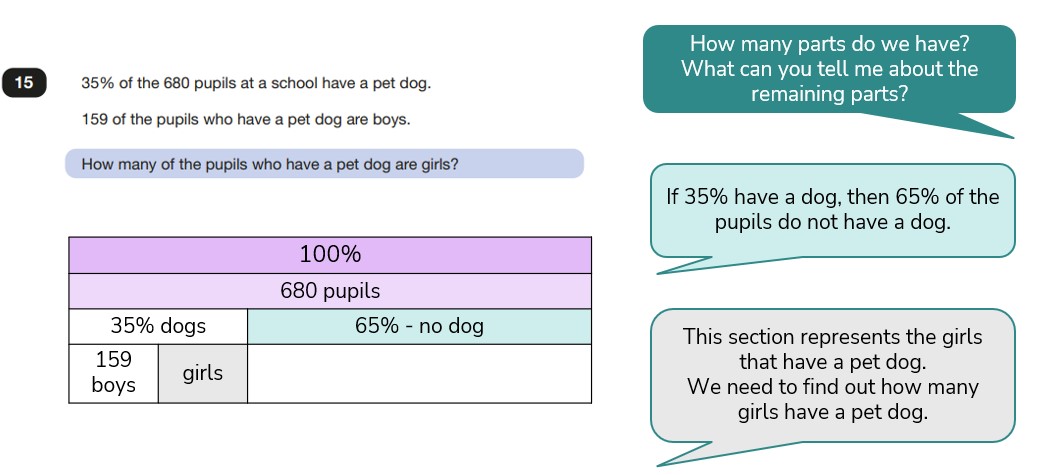
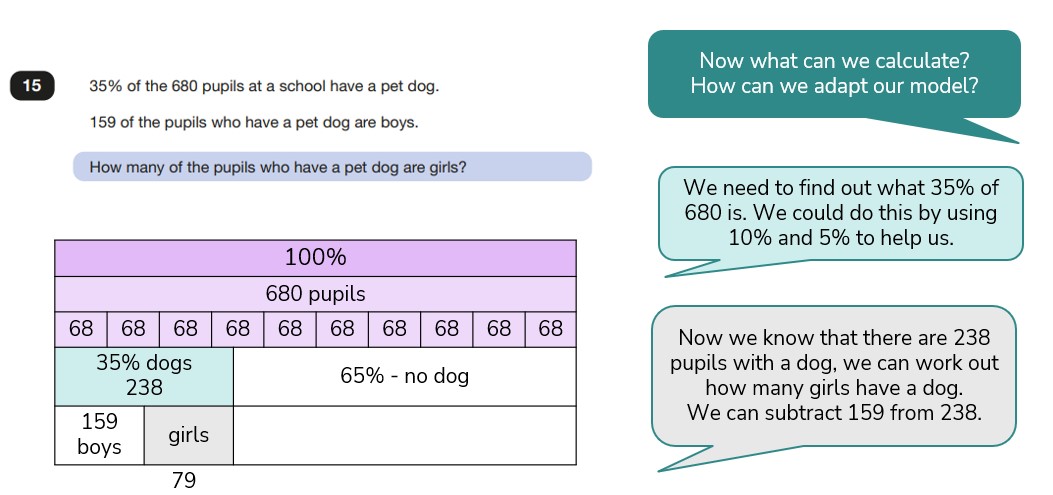
Solution
79 girls have a pet dog.
In this question, the bar model exposes the need to adapt models as scenarios progress and more information is discovered.
Question 18 – bar modelling to expose the need for addition, subtraction, multiplication and division

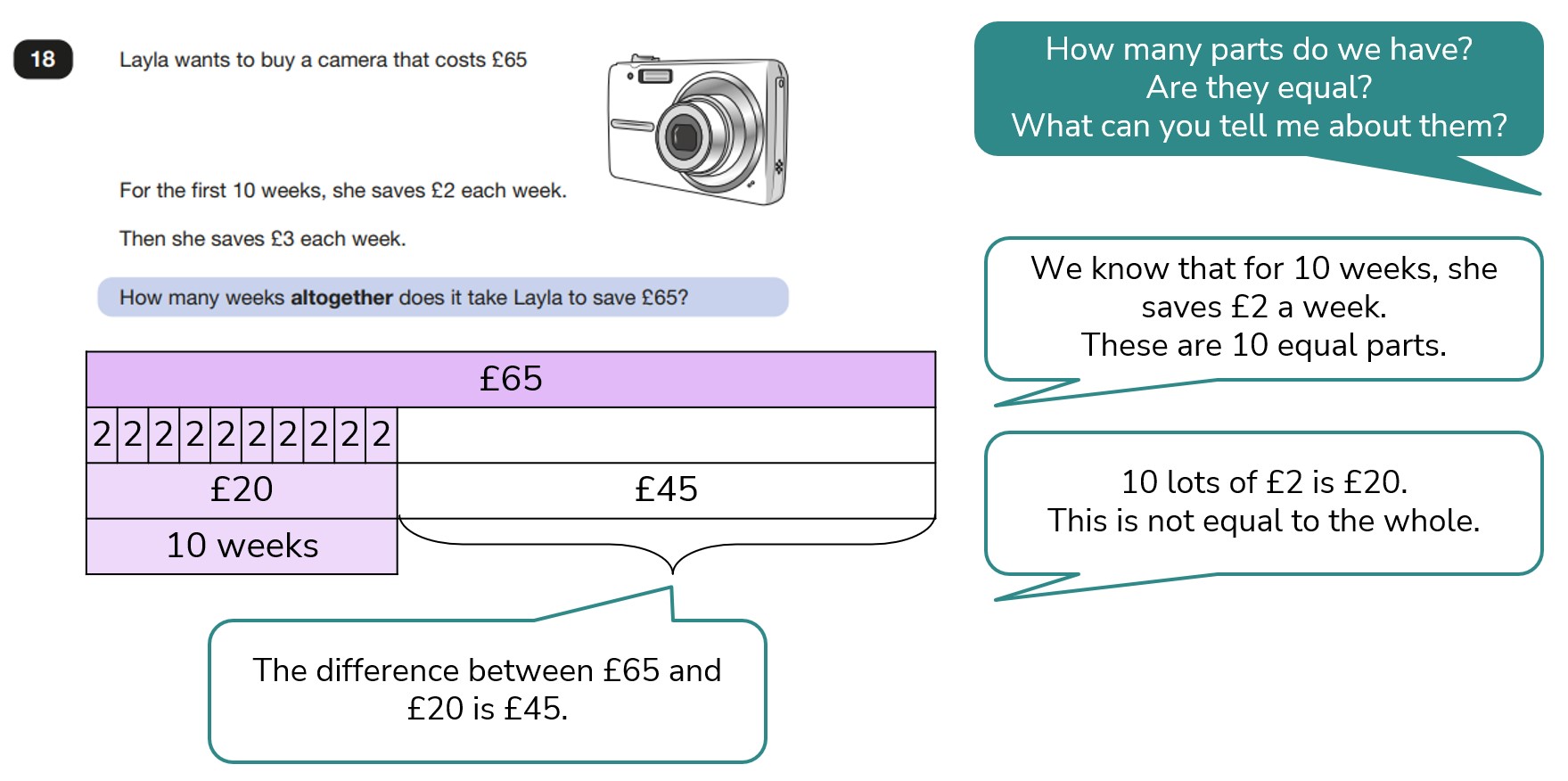
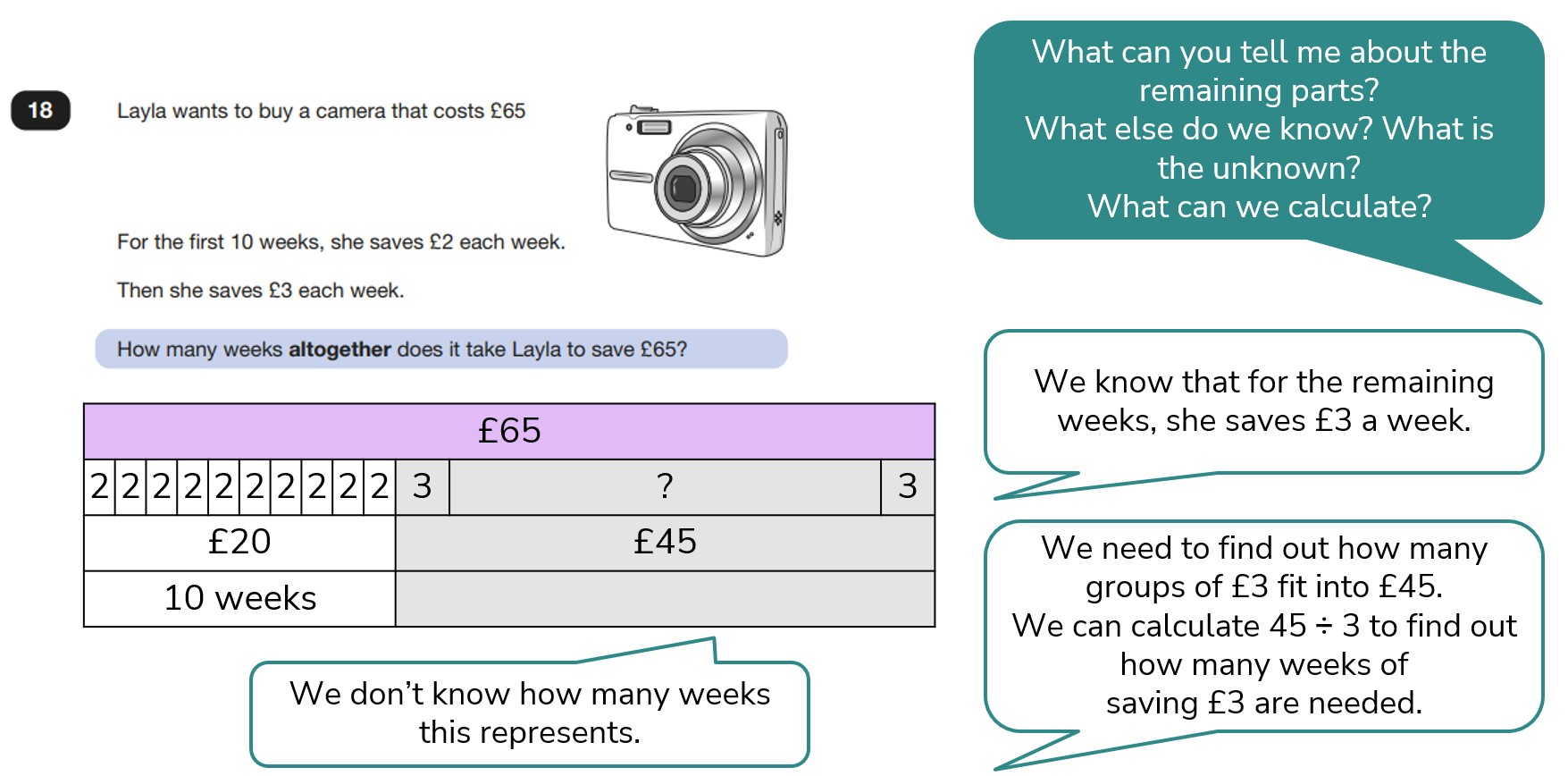
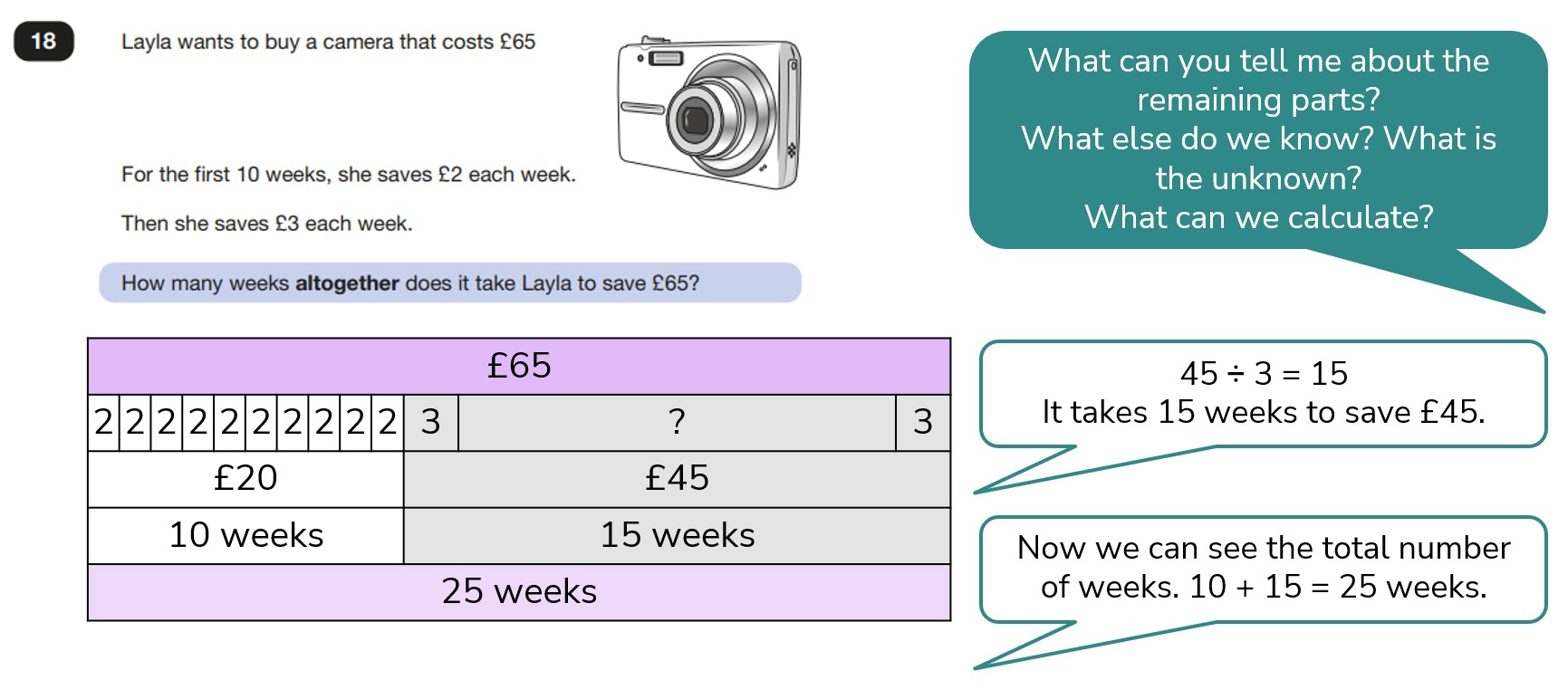
Solution
It takes Layla 25 weeks to save £65.
In this question, the bar model exposes models of multiplication (10 weeks x £2), subtraction (the difference between £65 and £20), division (grouping – how many groups of £3 fit into £45) and addition (augmentation – 10 weeks is increased by 15 weeks).
Question 21 – bar modelling to expose the need for mixed operations including fractions


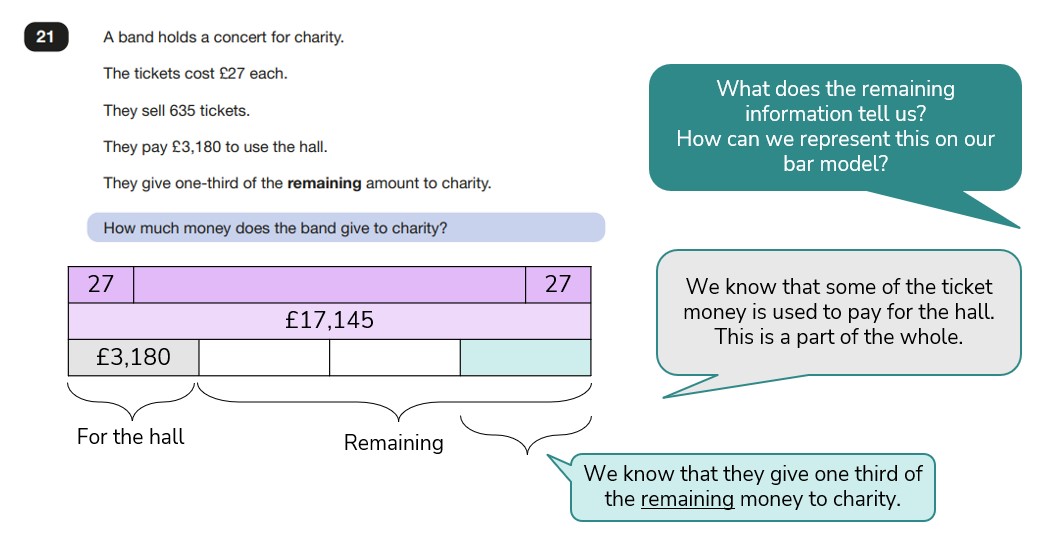

Solution
£4,655 is given to charity
In this question, we would not want the children to draw 635 groups of £27! However, through use of the bar model, they are supported to see the need to combine models of difference to find out the ‘remaining money’) and then to divide to find a third of this amount.
Further professional development
Our popular training is back this year in a new format.
Across four half-day sessions, we unpick tools for supporting children in meeting age-related expectations in maths using a range of different strategies, including the bar model!
Statutory assessment outcomes from schools who participated in this training in 2023/24 show that children made, on average, 6 months more progress than their 2022/23 cohorts.
This course is also available as part of the Year 6 teacher ticket package 2024/25.
Resources you may be interested in
Bar modelling in maths progression
- Download sample pages
- A combination of photos of concrete resources, pictorial bar models and abstract calculations brought together to exemplify how to solve problems from across the maths curriculum.
Year 6 maths gap finder: SATS preparation toolkit
- Diagnostic summative assessment papers for early identification of gaps
- Resources to support teaching and rehearsal of arithmetic and reasoning
- a suite of resources and analysis tools to identify specific areas of learning strength and development for pupils and classes when using any past SATs papers as practice (2016-2024).



