When considering end of Key Stage 1 teacher assessment judgements, evidence from the statutory KS1 maths tests will form part of the evidence base for each child.
Let’s delve into this statement from the Teacher Assessment Framework:
The pupil can add and subtract any 2 two-digit numbers using an efficient strategy, explaining their method verbally, in pictures or using apparatus.
Let’s consider this alongside question 17 from the 2023 KS1 arithmetic paper:
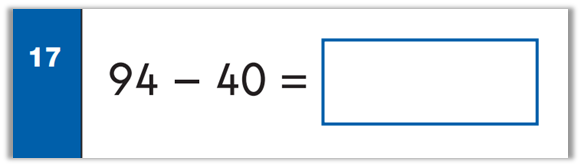
First, let’s see how long it takes to solve this using a one-to-one correspondence counting method…
I’ve sped up the video because it took 2 minutes 9 seconds to reach a solution.
This is plenty of time for a child using this method to become distracted in a busy classroom, resulting in an error being made. Plus, I’ve lined my dots up nicely – this is hard to do with so many to draw!
Possible points of error for children using this method:
1. drawing the incorrect number of dots
2. crossing out the incorrect number of dots
3. counting the incorrect number of dots remaining
If a child used this method and DID get the answer correct, would we be able to include this as evidence of this TAF statement being secure? I would suggest not as it lacks efficiency.
When analysing 2022 SATs papers with teachers this time last year, we came across plenty of examples of this very method being used for questions such as this:
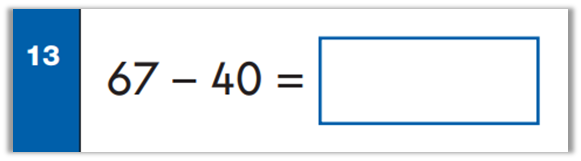
If this is a child’s ‘go to’ method, it becomes even more problematic when having to employ it in a wider context, where there are more things to consider.
For example, here, for question 23 in the 2023 KS1 reasoning paper:
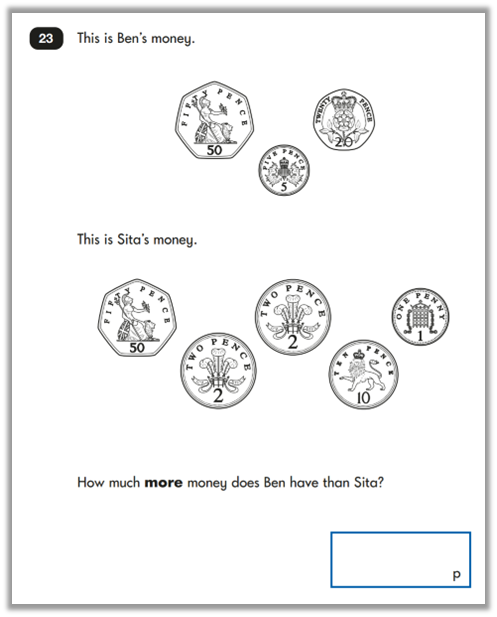
We could have a situation here where a child is having to:
- decide which coin set to start with (let’s say they choose to start with Ben’s)
- consider the value of each coin and draw the relevant number of dots (50 dots and 2 dots and 2 dots and 10 dots and another 1 dot)
- interpret that they need to find the difference, not add the two amounts (the word more has the potential to throw them here)
- cross out the number of dots representing Sita’s money (50 dots and 5 dots and another 20 dots)
- count each remaining dots to find the difference.
That is a HUGE amount of cognitive load and there are so many potential points of error.
What can we do to ensure children really can ‘add and subtract any 2 two-digit numbers using an efficient strategy, explaining their method verbally, in pictures or using apparatus’.
For questions like those considered so far, where there is no regrouping required to subtract, let’s explore some more efficient methods where a solid understanding of place value can be applied.
Another relevant TAF statement here is: ‘The pupil can partition any two-digit number into different combinations of tens and ones…’
Children need to understand ‘numbers within numbers’ – the parts and the whole.
Let’s revisit the first question above and explore some more efficient methods.
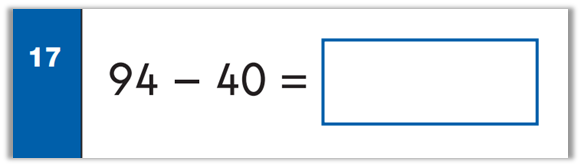
How can we get children to really see this?
Children can’t use the equipment in the test but this will support with visualisation of what is actually happening here when we take away part of the 94.
Connections between the abstract calculation, equipment and familiar part whole representations develop and secure understanding.
Language is important. Talking it through during modelling and independent practice ensures connection between the number names and the place value of each part.
We have ninety-four.
That’s 9 tens and 4 ones.
We take away 4 tens.
Now we have 5 tens and 4 ones.
That’s fifty-four.
Colours could be used to highlight the parts, alongside a speaking frame (as above):
This method could be represented pictorially:
Language remains important here too when considering the tens and ones.
These take much less time than drawing all the dots and they become even quicker once understood and the move can be made to purely mental calculation. Plus, there’s much less chance of error if number fact knowledge is secure.
Looking ahead to Key Stage 2
This is ok while understanding is developing but application to larger numbers would be cumbersome and it returns to being error-prone. Pictorially recording in this way should be a stepping stone to generalised understanding of how this method can be applied to larger numbers, taking into account place value:
Secure understanding of part whole relationships, tens and ones, and how to partition numbers flexibly will enable pupils to tackle problems across the curriculum in KS2 when they begin to work with a larger range of place values.
We certainly wouldn’t want to be relying on drawing dots to solve this one:

The HFL Education End of Key Stage 1 Mathematics Assessment Toolkit has been updated to include question level analysis materials and question-by-question TAF links for the 2023 KS1 SATs papers.
Contact the HFL Primary Maths Team at primarymaths@hfleducation.org to discuss in-school support for making accurate teacher assessment judgements at the end of KS1.
Professional development opportunities: autumn 2023
Join the HFL Education Primary Maths Team for face-to-face training at the Hertfordshire Development Centre in Stevenage:
Looking to develop pupils’ accuracy and efficiency in calculation as pupils move from KS1 to KS2 in your school?
Find out more about our Making Flexible and Fluent Calculators project, launching in autumn 2023.
To keep up to date: Join our Primary Subject Leaders’ mailing list
To subscribe to our blogs: Get our blogs straight to your inbox
References:
- 2018/19 teacher assessment frameworks at the end of key stage 1 for use from the 2018/19 academic year onwards
- 2022 Key Stage 2 mathematics paper 1: arithmetic
- 2023 Key Stage 1 mathematics paper 1: arithmetic
- 2023 Key Stage 1 mathematics paper 1: reasoning
Contains material developed by the Standards and Testing Agency for 2022 and 2023 national curriculum assessments and licensed under Open Government Licence v3.0.