
This blog concludes our series of blogs based on the KS2 2022 SATs papers. In the previous two KS2 blogs, we have explored the similarities and differences noted between the content and expectations of the 2019 and 2022 papers.
In this blog, rather than comparing papers and questions, instead we will focus on how we might use questions (or any reasoning questions) to teach and develop children’s reasoning skills.
You may have had a chance to read Nicola Adams’ blog published yesterday and unapologetically, what we explore here directly piggy backs on the ideas shared. If you haven’t had a chance to read it, please don’t be put off by the fact that it has a KS1 focus as the strategies and skills discussed are highly appropriate for KS2 children.
When working with teachers and children, it will come as no surprise to you that when asked about what type of questions are preferred, both groups often talk to us about strengths within calculations or questions presented in arithmetic formats but are less confident when tackling worded scenarios where increased reasoning is required. This isn’t a new finding. As my colleague, Siobhan King, writes in her blog from 2019,
‘Sometimes I think we can be guilty of expecting pupils to learn how to reason by osmosis.’
So, what practical steps can we take to support children with developing their reasoning and problem-solving skills?
It may be tempting to revisit the mnemonic RUCSAC to help children with the steps needed to solve problem solving and reasoning questions:
- read the question
- underline the key words
- choose the operation/s to use
- solve the calculation
- answer the question in context
- check that your workings and answer make sense
The problem with this is that children can very often slip up early on with these steps and so then the whole process stalls.
Instead of this approach, I will use the same approach that Nicola described in her KS1 blog, with a couple of tweaks for KS2, and exemplify it using 2 questions: 1 that is suitable for use with lower key stage 2 pupils and one more suited to upper key stage 2.
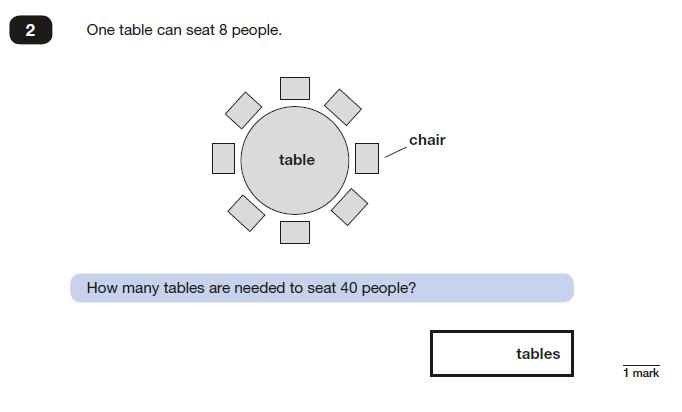
I have chosen the questions based on their content domain reference from the SATs mark schemes. Question 2 of paper 2 is referenced as 3C8/3C6 (multiply/divide mentally and solve problems using calculations).
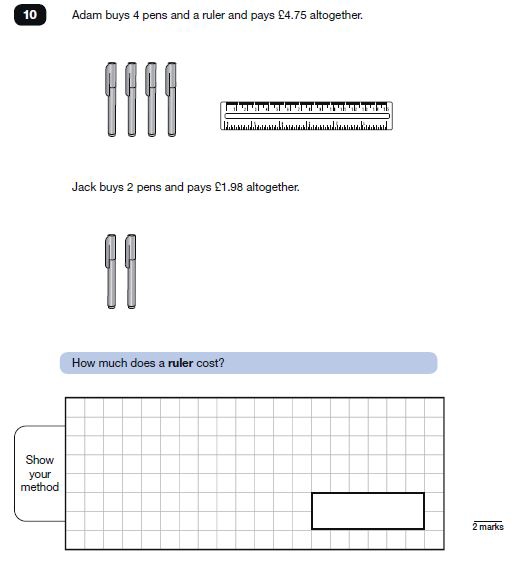
Question 10 from paper 3 is referenced as 5M9a/6A4 (solve problems involving measures and tackle number sentences involving two unknowns).
Just a quick caveat here – if you are using the content domain references to consider the pitch of specific questions, it is also useful to note that the complexity of the expected response can elevate the challenge of the question.
Step 1: Direct pupil focus
To begin with, remove as many distractions as possible from the question and decide what we want to draw the children’s attention to. This may depend on the chosen question as to what information you choose to start with. The important thing here is reducing possible anxiety and potential information overload. We want to slow the children down. In both the examples below, all text has been removed from the questions but sentence starters have been provided as a starting point to the question, ‘What do you notice’.
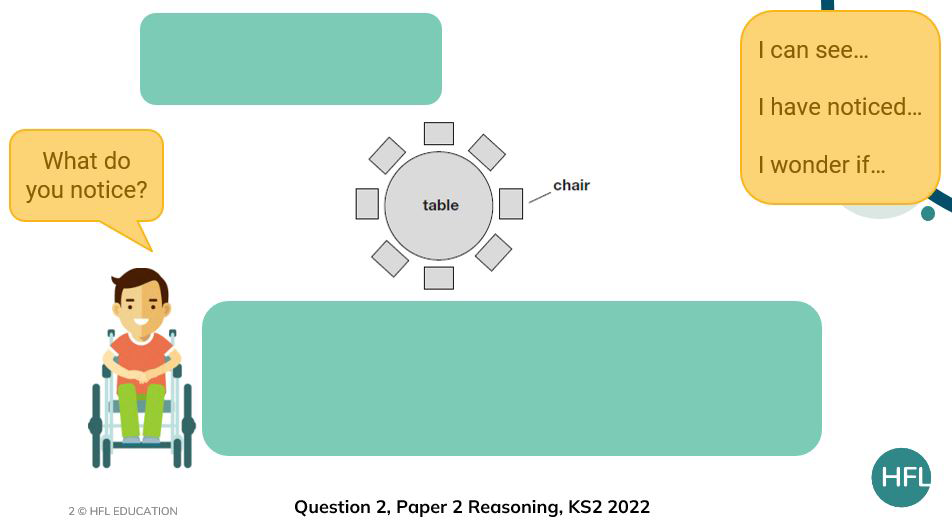
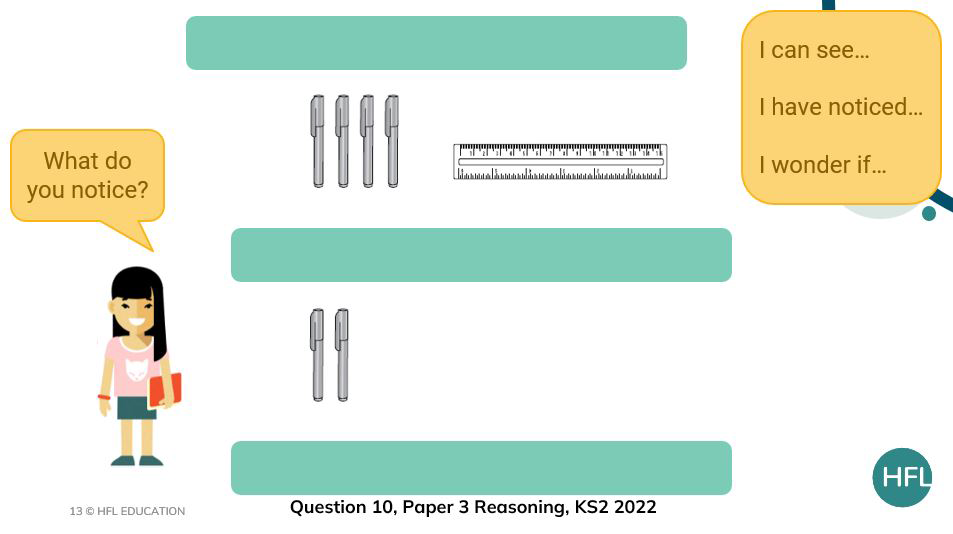
Children should be encouraged to discuss what they can see and what they notice in pairs, in small groups or as a class. Included here is also the sentence stem, ‘I wonder if…’ which could either be presented with the other sentence starters or be added after.
The initial observations may be simple and that is fine. We want all children engaged and talking and so any response goes!
Step 2: Refine pupil thinking
Following the initial conversations, we then want to encourage children to ‘filter’ and refine their thoughts.
To do this…
- provide a list of key vocabulary
- expect pupils to articulate their thoughts in full sentences
- ask pupils to listen to others to see if they can add more details or be even more precise
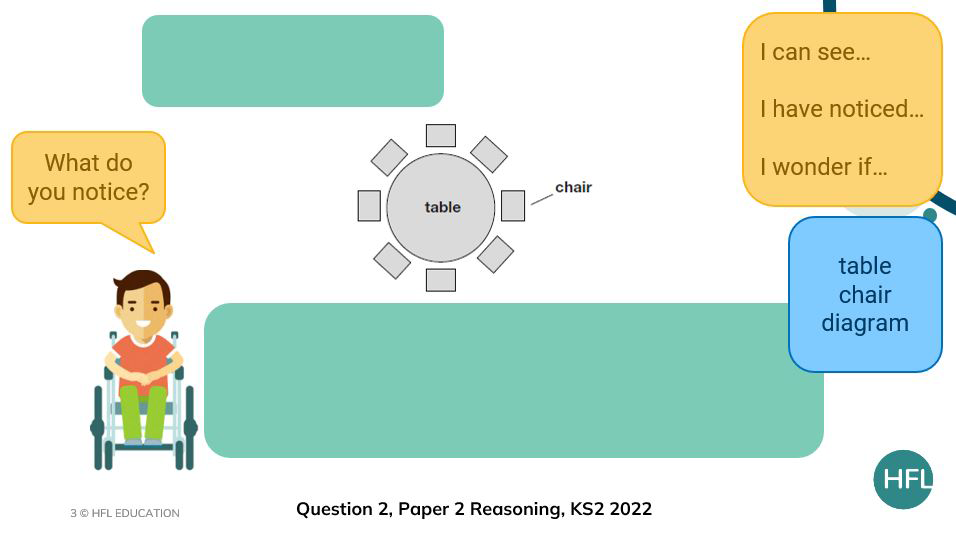
With question 2, the vocabulary list may be simple and draw attention to the fact that a diagram has been provided as opposed to literal pictures of tables and chairs.
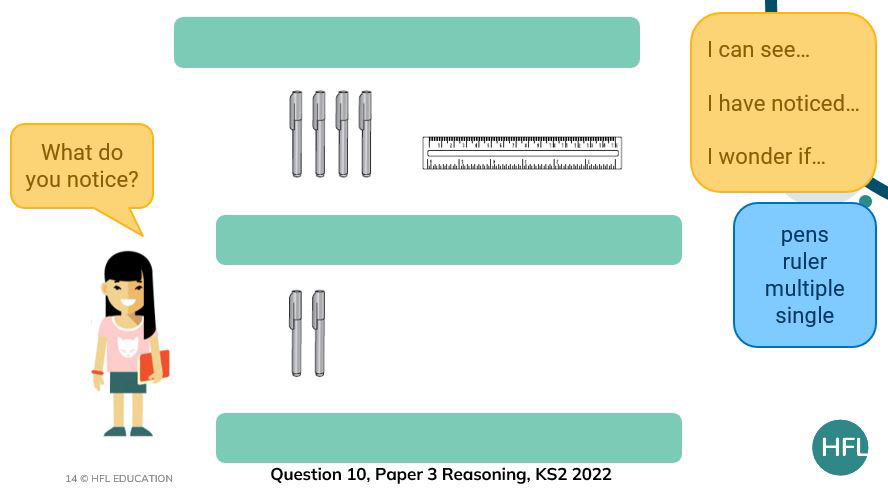
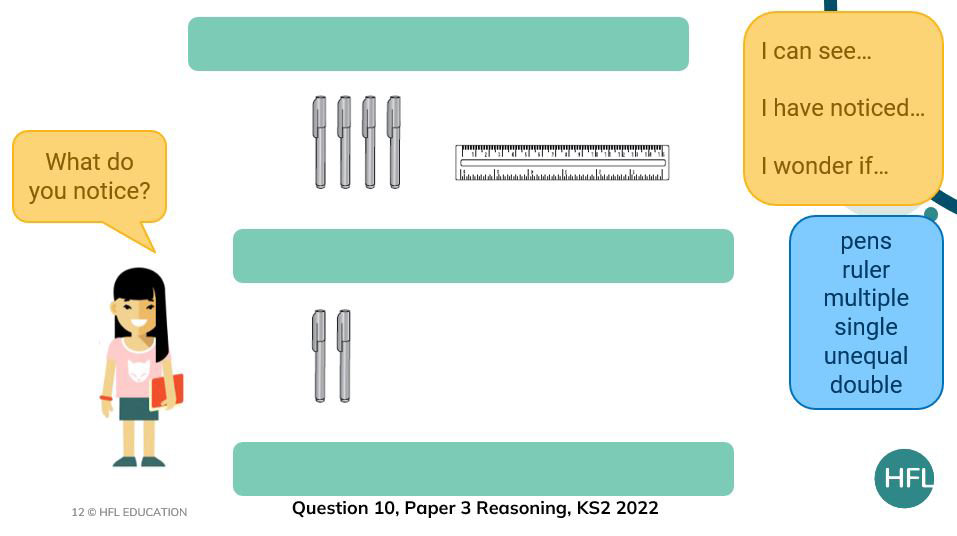
With question 10, two examples have been provided to show how the vocabulary could be layered during pupil discussions.
First, I would want them to consider simply what they can see in both the top and lower image and then I would want them to start comparing the images.
Do they notice that there are double the amount of pens in the first image than the second?
Can they talk about the unequal groups that they can see?
This will be important later when the question is finally revealed and so these are points that I want to draw attention to beforehand.
Step 3: Build on what they know
To do this…
- reveal information from the question bit by bit
- after each reveal, ask the pupils, ‘What do you know now?’
- ask pupils to suggest any additions to the vocabulary list
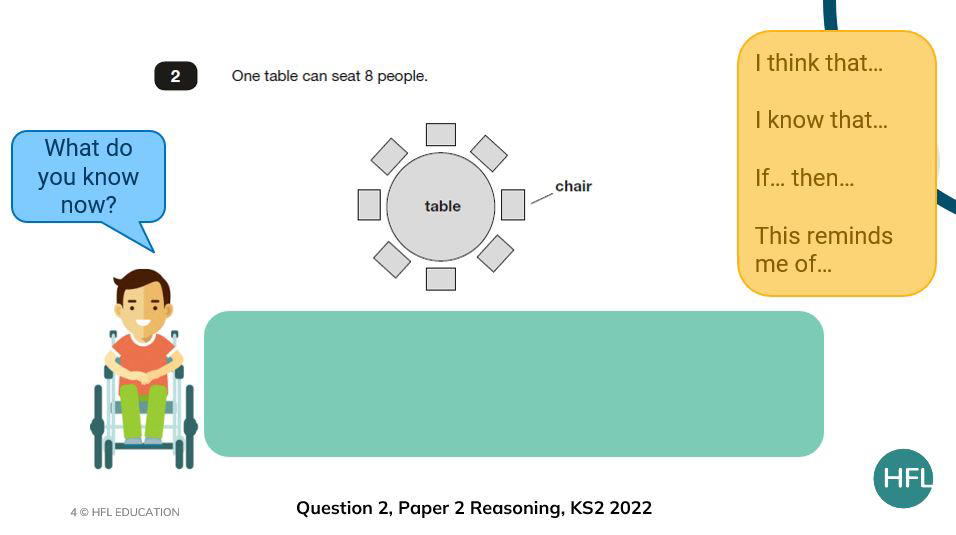
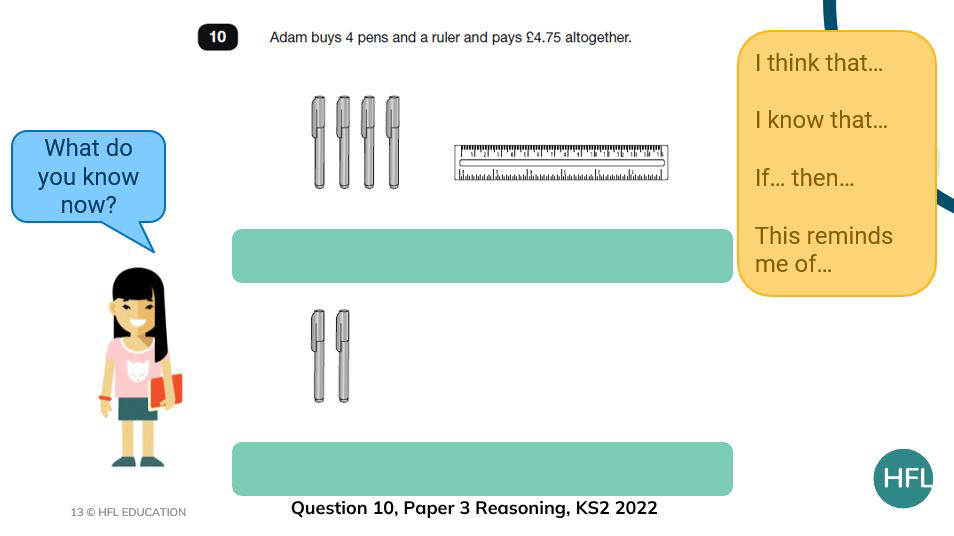

The revealing of information is always my favourite part of the teaching sequence especially where there is additional information to reveal such as in question 10.
Asking the children what information they would like to know or find out encourages them to think about associating different information with parts of the question.
For example, at first, we know how much the 4 pens and the ruler cost altogether but it would be very useful to know either the cost of a pen or how much the ruler costs as we are dealing with two unknowns here. I tend to ask the children if they are ‘ready for more information’ to build the suspense!
We can also continue to use the initial sentence starters but also encourage children to make links with real life experiences and other learning by including the stem, ‘This reminds me of…’.
For question 2, children might be thinking about sitting in the school dining room or thinking about equal groups for games in PE as examples. Again, this focus is important as later on the children will need to be thinking about equal groups to solve the actual question – they just don’t know it yet!
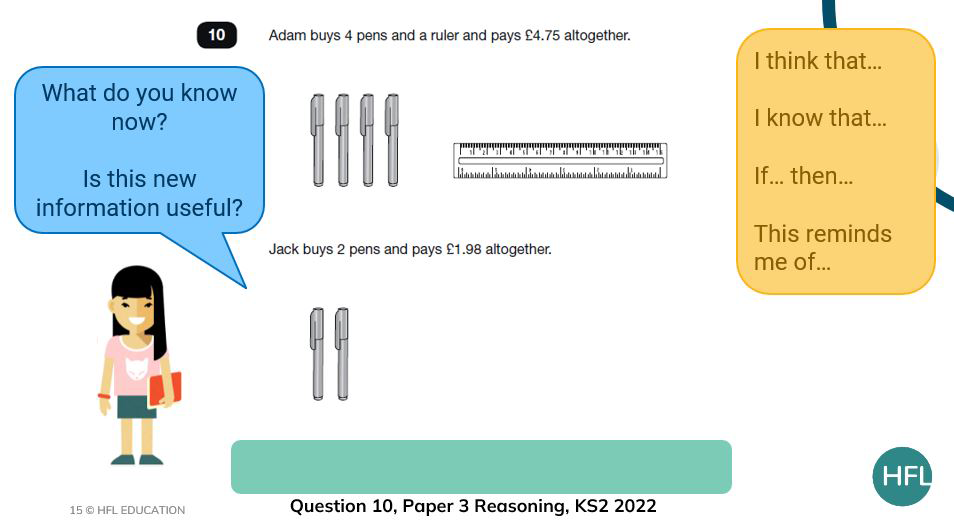
Asking the children to compare the initial information with the added information and to assess the usefulness of this new information adds to the challenge for those who are ready for it.
Step 4: Consider ‘the question’
To do this…
- BEFORE revealing the question, ask pupils, ‘What could the question be?’
This allows you as the teacher to assess whether the pupils are considering the likely structure of the problem. Using a vocabulary bank, whether this is the same as the initial bank, or with some additional words added can be a useful opportunity to further refine children’s thinking.

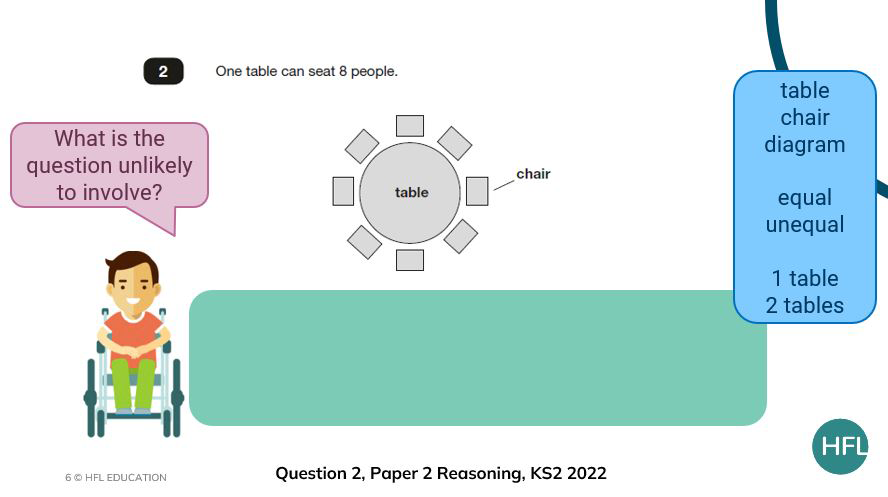
In this example, are the children considering questions that would involve multiplication, division, and equal groups such as in the scenarios they may have been talking about using the sentence stem, ‘This reminds me of…’
If a child came up with a question such as, ‘How many children could be seated at the table if we took one chair away’ (which should still be a valued question), this would tell us that they have not considered the vocabulary of equal and unequal in the word bank nor the mathematical domain that this image or related situations would allude to.
Furthermore, asking the children to consider what is not likely to be included in the question provides a challenging opportunity for children to think about the choices that they have made when considering this question.
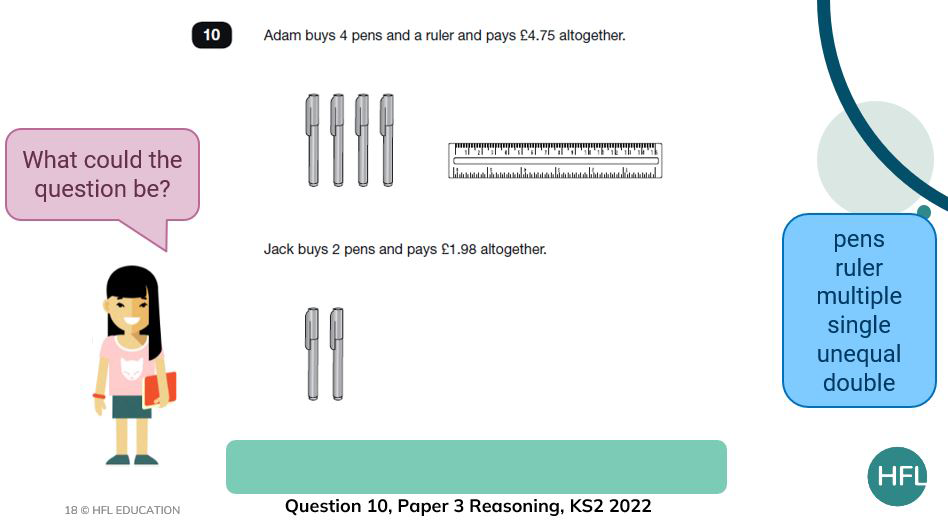
In this example, children might suggest finding the total cost of 6 pens and 1 ruler which, again, would be a valid and reasonable question and could lead to an interesting discussion. For that question, would we need to know how much the ruler costs on its own?
Step 5: Reveal ‘the question’
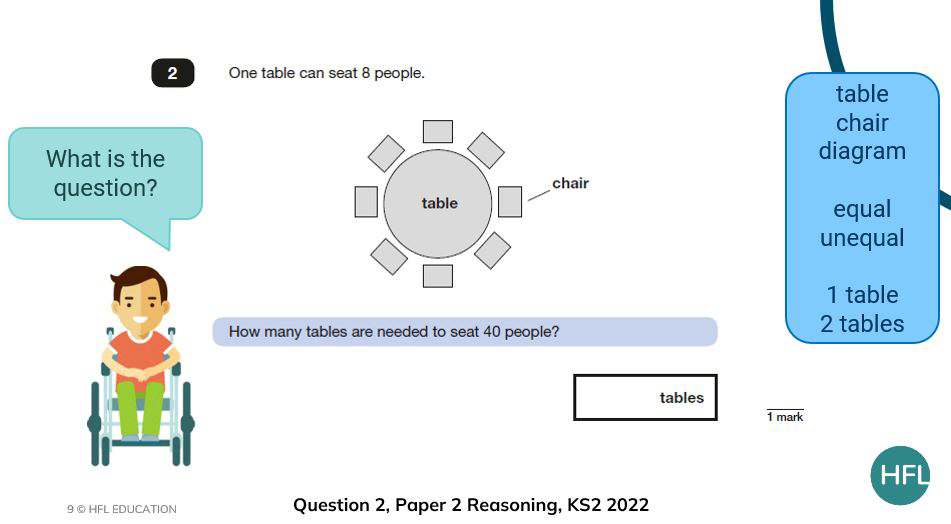
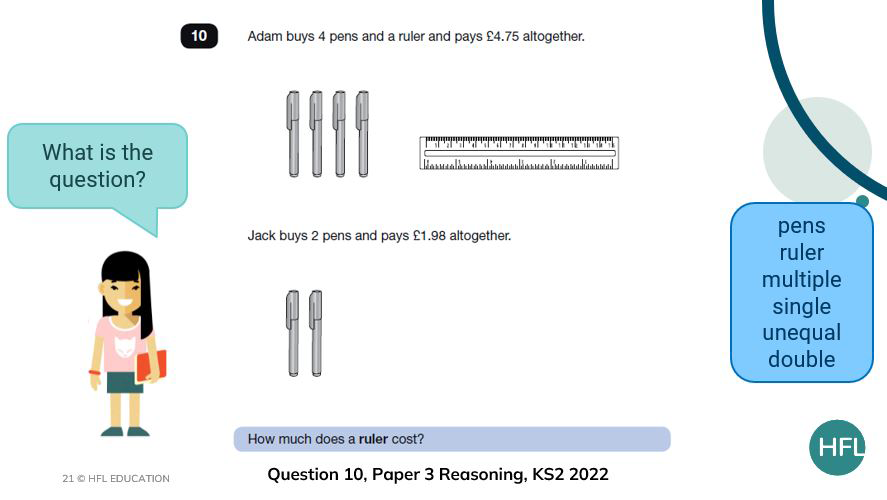
To do this…
- ask children to read the question aloud. Perhaps read it aloud as the teacher and then ask children to repeat it back
- ensure children understand all of the words within the question and link this to the model and vocabulary list if needed
Step 5: Reveal ‘the question’ – a simpler example
It may be that you want to take the opportunity to simplify the question when you first reveal it to encourage all children to access it and not be put off by the numbers used in the ‘actual’ question.
In the example below, instead of seating 40 children, I want the children to firstly think about seating just 16 children. I want them to do this to draw attention to the fact that we are working with equal groups of 8 and connect division and multiplicative scaling.
I would tie this in with the next step – representing the problem – and explore how we could represent this by annotating the diagram, drawing a bar model or listing calculations for example.
From here we could then ask them what if we have 20 children? Do they consider the fact that 2 tables will be needed for 16 children but there will be 4 children left who will need another table. You could then reveal the actual question and children could apply this thinking to 40 children.
Is the final question easier or harder than when we thought about 20 children?
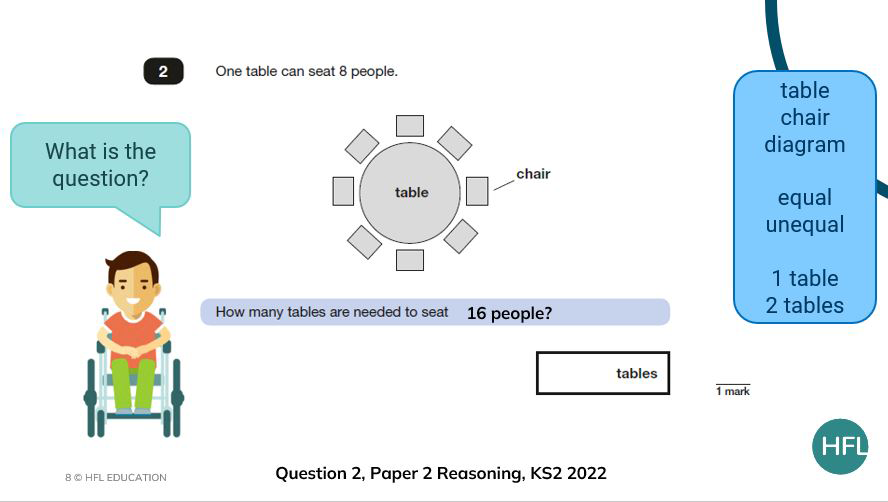
Step 6: Represent the problem
By this point, we are beginning to consider the calculations needed to solve the problem at hand. For some, considering one representation is challenging but important in supporting them to ‘choose’ the operations needed. Whereas for other children, encouraging multiple representations can prove challenging as we may be asking them to think further than their default method.
To do this…
- ask the children, ‘How could we represent the problem?’
- be specific about representations – ‘What would it look like as a drawing?’, ‘What would it look like as a bar model?’, ‘What would it look like as a calculation?’
- ask the children to compare their representations to a partner’s. Can they see where each part of the problem is shown? Can they explain their representation or model to someone else?
- support children to make connections by modelling and comparing representations. Can they match bar models to appropriate operations?

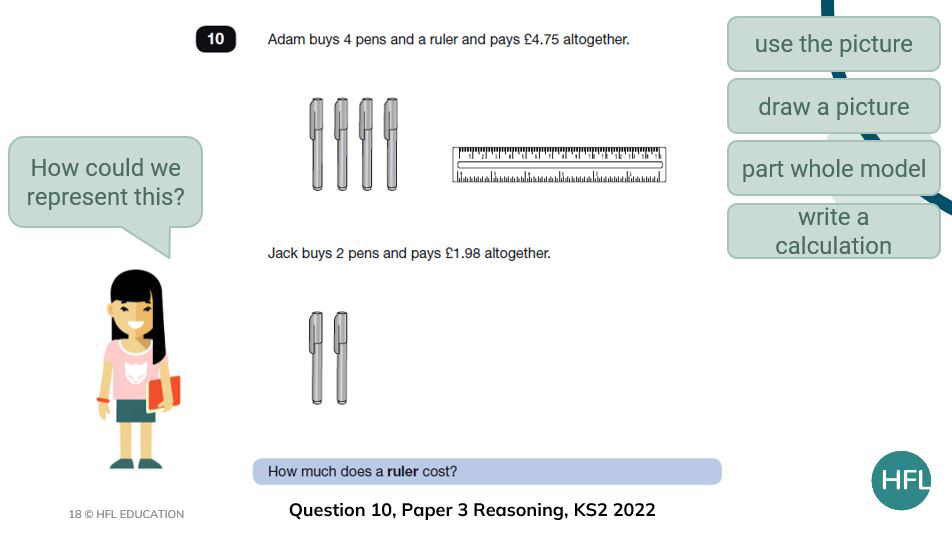
So far, there has been an emphasis on talk and discussion and now, it would be useful to ask children to use jotters or mini whiteboards to draw their representations. They can then use these to explain and compare with friends as well as providing adults with opportunities to ‘mark’ over the shoulder and draw out misconceptions which could then be shared with the whole class.
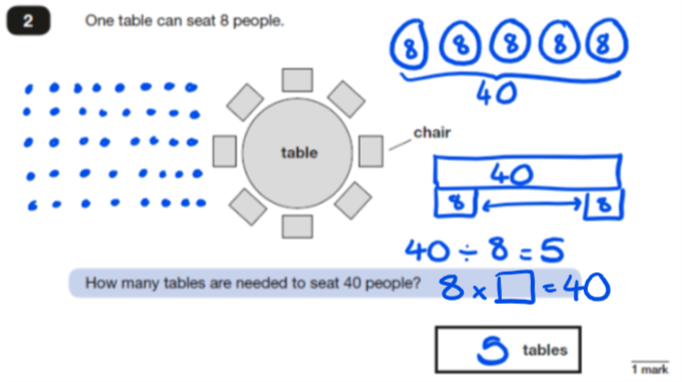
Some children might represent the problem by using an array and 1:1 correspondence and skip count in 8s to keep track of when they reach 40 people. These children should be encouraged to identify the group size (8 people) and then number of groups (5 tables) to check that they are using the correct structure for the scenario.
Children might also recreate the table image by drawing groups of 8 where they have moved away from requiring 1:1 correspondence while other children might represent the problem using a grouping structure bar model, identifying that they are looking to find how many groups of 8 can be made from 40. Children may then use these models and representations to write an equation to show which operation they will use to solve the problem.

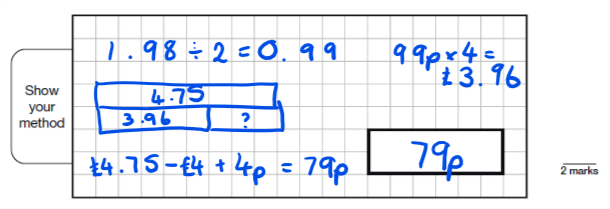
Children might choose to draw on the images provided before writing any equations such as the first example where they have identified that to find the cost of 4 pens, the cost of 2 pens could be doubled before subtracting this from £4.75. Alternatively, they may choose to draw and write straight into the given answer box if they have been provided with copies of the question.
As when the children were discussing what the question could be, all of the children’s representations and models should be shared and celebrated.
Step 7: Find the answer
To do this,
- ask children to identify on their representation where the answer is shown
- ask, ‘Have you identified the operation needed?’
- ask, ‘Are there different ways of finding the answer?’
- ask, ‘What could go wrong when you are finding the answer?’


Again, at this point children should be given the opportunity to write down their answers including any jottings or drawings that they make alongside. Examples could be explored under the visualiser, and these could include successful examples of workings out as well as any errors which can then be discussed as a class.
For example, for question 10, do children double £1.98 using equal sum (£2 x 2 – 4p) before subtracting this from £4.75 or do they use a less efficient method of finding out what one pen costs and then multiplying by 4 before subtracting?
For question 2, which children choose the correct operation and divide 40 by 8 but skip count incorrectly or use an inefficient method for division?
Step 8: Check the answer makes sense
How often do the children down pens or move on after they have written their answer?
How many times do they groan at their ‘silly’ mistakes?
This step provides the perfect opportunity to slow down and check that answers make sense.
To do this…
- Consider all the information the pupils used while problem-solving (the image, the vocabulary provided, the key words in the question, any models or drawings)
- Ask the children to complete the sentence, ‘The answer is … because …’
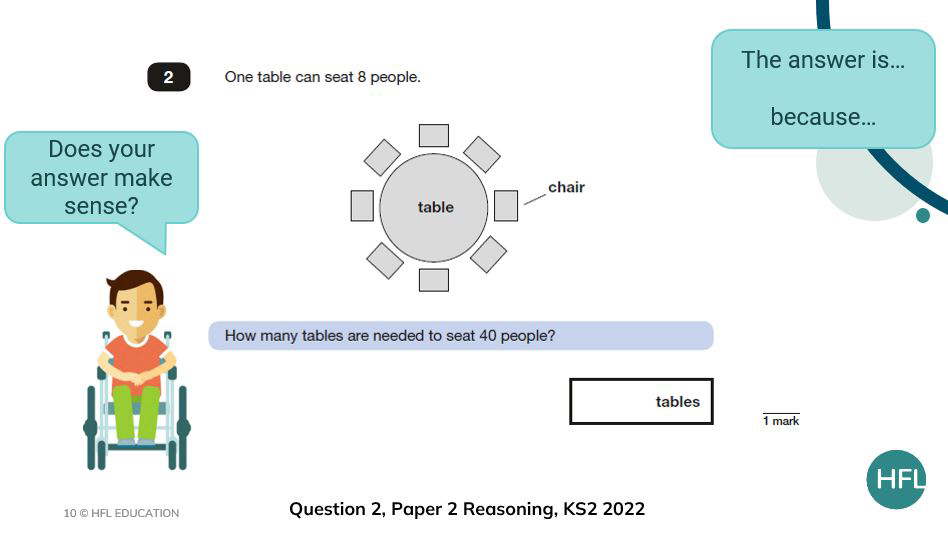
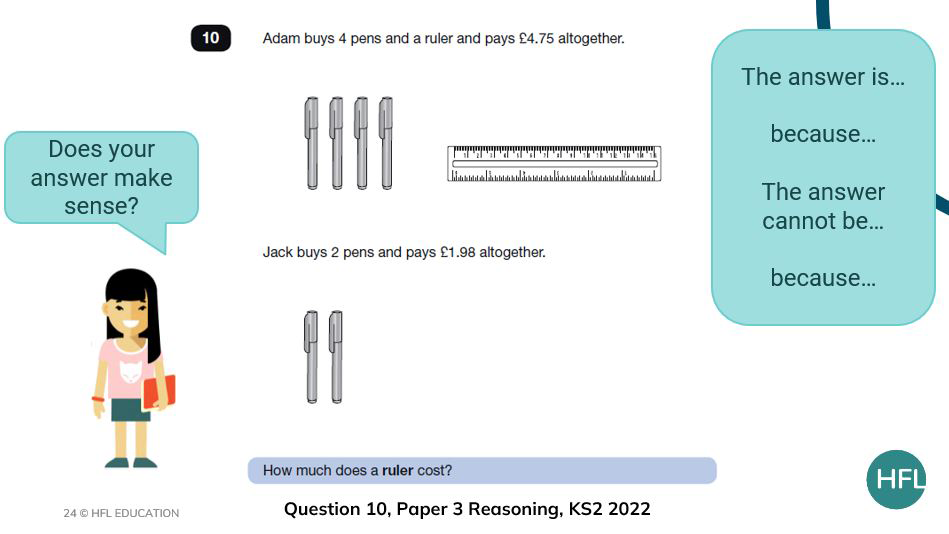
Adding in the additional sentence starter ‘The answer cannot be… because…’ allows misconceptions already discussed to be used by the children or the children to consider their own misconceptions whether they have made an error or not.
Further exploration – getting the most out of the question!
If appropriate, further exploration of the scenario could take place.
For example, children could go back to their initial responses to, ‘What could the question be?’ and answer these. Are they easier or tricker than the actual question?
Or a variety of ‘what ifs’ could be delved into.
For example, what if the answer to question 2 was 7 tables? What could the question be? How many different options are there?
Or for question 10, what if the unknown was different? What if we were told how much pens cost and how much a ruler costs but we had to find the change from £5 for 4 pens and a ruler? Or what if division was banned for question 2; how else would you solve it?
There are so many possibilities for continuing to explore the questions.
Developing independence in solving problems
What we would like to see over time is pupils developing more and more independence in using skills and ideas from these steps to solve problems themselves.
When planning a sequence of teaching and learning, refer to the SATs papers for questions that it would be opportune to drop in, either in the way outlined above, as a teaching opportunity, or as an independent or paired discussion task to allow assessment of how pupils tackle them.
This will also provide crucial information about which of the steps the children tend to find the trickiest. Is it articulating what they notice in full sentences? Is it drawing a model to support with choosing an operation? Or is it something else?
Whatever the sticking point, this can form the focus of teaching going forward.
There are plenty of past papers from which to choose questions and these can be accessed here:
- Key stage 2 tests: 2022 mathematics test materials
- Key stage 2 tests: 2019 mathematics test materials
- Key stage 2 tests: 2018 mathematics test materials
- Key stage 2 tests: 2017 mathematics test materials
Further reading
How to use the KS1 reasoning SATs papers to teach children problem-solving skills
References
- Mathematics test framework: National curriculum tests from 2016 for test developers
- 2022 Key Stage 2 mathematics paper 2: reasoning
- 2022 Key Stage 2 mathematics paper 3: reasoning
Contains material developed by the Standards and Testing Agency for 2016 and 2022 national curriculum assessments and licensed under Open Government Licence v3.0.



