
Siân Muggridge is a Specialist Advisory teacher for Vision Impairment (VI). Over the past few terms, Gill Shearsby-Fox and Siobhan King from the Herts for Learning Mathematics team have been lucky enough to learn from and with Siân to continue to develop understanding of how to best support pupils with vision impairment in their mathematics learning. We have been able to discuss priorities and curriculum adaptations that support success and Siân’s practical adaptations of resources and learning opportunities know no bounds! It is wonderful when you meet someone who is similarly passionate about successful mathematics learning for all children, and we are delighted that she has agreed to share some of her expert insight and tips in this guest blog.
How many numbers do you think you have read today? Your clock as you got up? The expiry date on the milk carton? The numbers on the remote control as you turned the telly on? Three examples and we’ve yet to leave the house.
Now imagine if you never saw numbers in the environment, that you never notice the numbers on doors or how they go up in twos, or that you can’t tell how many apples are left in the fruit bowl without going and physically touching each one. This is the experience for many children who have a vision impairment.
My name is Siân and I have been working as a Specialist Advisory teacher for Vision Impairment (VI) for seven years. I first became interested in the relationship between vision impairment and maths when I met Nabeel (not his real name) in 2015; a Year 4 pupil with a moderate VI and a physical impairment. Nabeel was articulate and doing well in English but, despite having a high level of support, adapting his resources, and using a special magnifier to see the board, he seemed to be really struggling with maths – even simple activities such as adding two numbers appeared to be beyond him.
When we dug a little deeper, it appeared that Nabeel had serious gaps in his knowledge: he wasn’t secure with place value, he didn’t realise that numbers had a set order (ordinality) and he struggled with manipulating shapes and understanding pattern (for example he struggled to put 2 Numicon pieces together). It was evident that there were gaps in Nabeel’s maths concepts that needed to be plugged if he was going to make progress.
We therefore set some challenges that Nabeel could do each morning independently, focussing on developing his understanding of ordinality, place value and using manipulatives effectively to solve problems (see below). It helped to plug some of the gaps in Nabeel’s understanding and, importantly, his confidence in maths grew.

As I continued working with more pupils with VI, I found several of them struggled with maths and, those who had little or no vision and were learning through touch (braille), often found maths the hardest subject to master. Why should this be?
Well, it’s not a difficult question to answer when we think about it – maths is a highly visual subject that relies on the ability to sequence and organise ideas visually (ICEVI 2005) and most children will often begin this journey before they start school.
Children with VI will often lack these incidental learning opportunities (like the ones mentioned at the start) that their typically sighted peers have accessed. Indeed, it has been argued that concepts such as subitivity, cardinality and ordinality can develop before children receive any maths instruction (Van Der Heyden 2011). This is not to say that early maths concepts cannot be ‘caught up’ but that we need to know where the gaps are. For instance, it’s hard to teach cardinality if you don’t yet know what the concept of same and different is.
Indeed, it took me 3 months attempting to teach one-to-one correspondence with a little girl who had no vision by counting objects from one pot into another. We could not get beyond counting 2. Why two? She had two hands and could deal with the concept of two. However, as soon as she put that object down, she didn’t really understand that it still existed and therefore started counting again. She needed to develop the concept of object permanence (that objects still exist even if you can’t perceive them) before she could develop counting past 2. Once she understood that concept however, she absolutely flew and could count as many beads or bricks as I could fit in the pot.
But why am I going on about this?
Vision impairment is a low incidence condition, which means you can go through your whole teaching career without meeting a child with VI – particularly one who uses braille. However, there will be plenty of children who may have, or had when younger, difficulties processing visual information and there will be some children who have missed those early incidental opportunities for different reasons. To support mathematical success for all pupils, I have identified some helpful tips which could help to make maths a bit more multi-sensory and, dare I say it, a little bit less visually complex.
Tips for nursery/reception
Children’s vision continues to develop until about the age of 7 so it’s not unreasonable to think that some children in Reception will be less adept at processing visual information or using their distance vision. You may also find some children are being patched to try and strengthen the vision in their ‘weaker’ eye – obviously these pupils’ vision is reduced while they are being patched.
- consider the environment: a patterned tablecloth can be tricky when trying to complete a visually complex task. You can stick a bit of black sugar paper or a mat down to provide a clear, high contrast background
- sometimes, providing a lipped tray to ensure that resources stay in the right place can help. The one below (Picture 1) has red grip tape so that objects stay in place, and it provides a high contrast background

- a lap tray can be a very useful resource for a pupil on the carpet. The one pictured below is £9 from Ikea

- there are lots of incidental learning activities in play – some children may need more directing to them than others. If you’re singing 5 little ducks, have you got the ducks in front of the pupil who needs a more multi-sensory way of learning?
- concept books can be useful for reinforcing ideas that pupils haven’t quite grasped. In the examples below (which I promise didn’t take very long to make), the key factor is that concepts have been exemplified, enabling generalisations to be made. A circle can be a coin, bottle top, a badge. It can be big or small. Two things can look the same or different. They can feel the same or different or they can sound the same or different


- be careful when reviewing concepts such as big and small, that you are not confusing with other concepts such as colour or shape. I once watched a lovely session with a TA and two pupils sorting leaves into big and small – she picked a big brown leaf and said big, then a small green leaf and said small. Her third leaf was a big green leaf. She asked the pupils whether the leaf was big or small. They both said small!
- one-to-one correspondence: counting images on a sheet or objects in a line can be tricky for some children. If you find pupils struggling, try counting from one pot to another; even better if the object makes a sound when dropped into the next pot
- it can also be helpful to add some tactile markers for counting and addition. This can give some sensory feedback when counting. Drawing a high contrast line around images/gems (Picture 6) or using a high contrast background (Picture 7) can also make them easier to discriminate


Tips for Key Stage 1
Of course, all the suggestions for Early Years will work as well for pupils in Key Stage 1, but it might be more evident that some pupils are struggling with maths concepts. One of the major areas of challenge that we have found for pupils who are learning through touch (with braille and tactile diagrams) is how many activities focus on subitising (recognising an amount by looking at it).
- physically explore part and whole to see how numbers live within numbers. If you learn through touch, then you are always working from the part to the whole and you can never just ‘know’ how many objects or images are in the set until you have counted each one individually. One way of getting around this is to focus on using fixed patterns such as Numicon. Pupils learn to recognise the Numicon shapes and can compare sizes, learn about odd and even numbers, order numbers and begin to explore number bonds
- many pupils struggled with manipulating the Numicon shapes and fitting them on the white board as they would fall off. Children with difficulties with binocular vision or fine motor skills may also find this tricky so a colleague made a special board from MDF and dowls (see picture below) that allowed them to explore number bonds to 10 with Numicon. Having the longer dowls meant that they could also be used with beads without them falling off

- tens frames: there are many ways to represent number bonds or amounts on a tens frame. Magnets, washers, and a bit of card can make a handy reusable tens frame.

- having found that some pupils find it tricky to use number beads, I found two simple adjustments that seemed to really help. Rather than using an abacus, Rekenrek or bead string, where the beads could easily slip back and forth, I found that this handy device (picture below) worked well as the beads stayed in place. I had to look it up and it’s a golf stroke counter and, of course, you can make your own – just google it!

- another adaptation was to add washers in between the 10 beads on a hundred bead string (picture below). This way, rather than counting each bead individually, the pupils were able to identify the tens quicky and count on from there

- younger pupils may need larger print size when they are becoming familiar with numbers and operation signs. Have a look at early reading books and you’ll see most of them are in font size 28. We found this large 100 square (picture below) had a variety of uses, for example, ordering numbers, exploring number patterns or as a physical number line
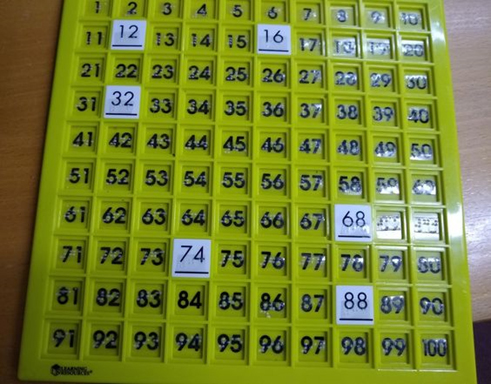
- it’s worth being aware that some “helpful” visual representations might not be that helpful for all the children in your class. It might be necessary to reduce and simplify the visual information – changing pictures into shapes or even putting a thick black line around pictures can often just make them easier to identify
Tips for Key Stage 2
I have found that Key Stage 2 can often be more challenging as the use of manipulatives tends to be reduced and the organisation of mathematical ideas becomes more set (e.g., place value, column addition, formal division methods). However, there are still things that I have found useful:
- keep manipulatives going for a bit longer. It’s very hard to understand concepts such as regrouping (exchanging) if you can’t see the physical exchange process. I was teaching a pupil, who uses braille for learning, how to do column addition; we had to first understand the concept of a column and then what was meant by exchanging a ten for ten ones. We practised this multiple times before she was confident to do this ‘in her head’
- think representational rather than pictorial. I’m sure everyone is familiar with the concrete, pictorial, abstract (CPA) approach to maths but what happens if the pupil you’re teaching can’t see the pictures, doesn’t process visual information very well or can’t easily draw their own images? I would suggest the need to reconsider the pictorial and think more representational – for example, instead of using a worksheet that used different coloured place value counters to represent 1000s, 100s, 100s and 1s, I created some different shaped magnets that could represent the counters (picture below). It saved huge amounts of time adapting resources and allowed the pupil with VI to work alongside her typically sighted peers

- reduce the visual processing. I once observed a maths lesson focussing on place value where the pupil with VI meticulously copied out the place value chart from the board but unfortunately, she took so long, she had missed the explanation and didn’t understand what to do. Having a ready-made chart that could be written straight onto can be very helpful for pupils who take more time to copy from the board. I made this one (picture below) for the student I had observed as she found it very difficult to recall which way digits moved when they were multiplied / divided by 10 or 100
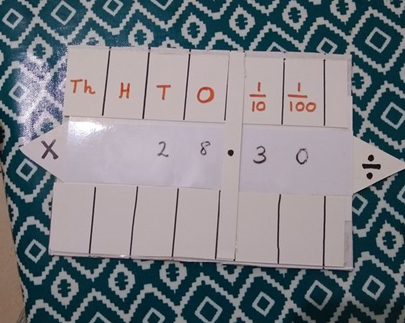
- be aware that pupils who rely heavily on their hearing and do not have the incidental opportunities to see numbers will often write numbers how they are said. For example, 136 may be recorded as 100306. These pupils need more opportunities to read and write numbers
- ensure that font size is not too small and there is enough space for working out calculations. Unlike printed text, numbers and operations signs have no context to draw on and, if you make a simple error such as mistaking a 3 for an 8 or a + for a ÷ then your answer will be completely out! There seems to be a tendency (especially for homework) to provide teeny weeny printed calculations. While this might be beneficial for the photocopying budget, it may not be so good for the pupil who struggles to differentiate numbers and symbols
- highlight the operation signs. Even if print is large enough, some pupils may struggle to differentiate between operation signs so use different colours to highlight the different operation signs and to make them easier to identify
- beware working walls! (picture below) They can be a fantastic way to record lots of different methods of solving a maths problem but can be very confusing for any pupil who finds it hard to process visual information. For some pupils, it might be helpful to have methods laminated on cards or magnetic boards in front of them that can be referred to
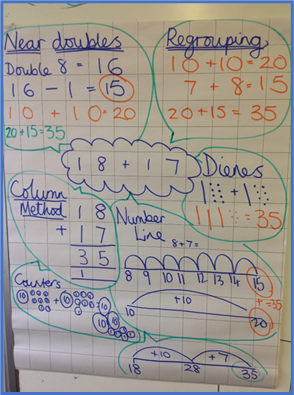
It might also be useful to focus on 2 or 3 preferred methods rather than attempting to use all of them – some methods may be less helpful because they are visually confusing (picture below).
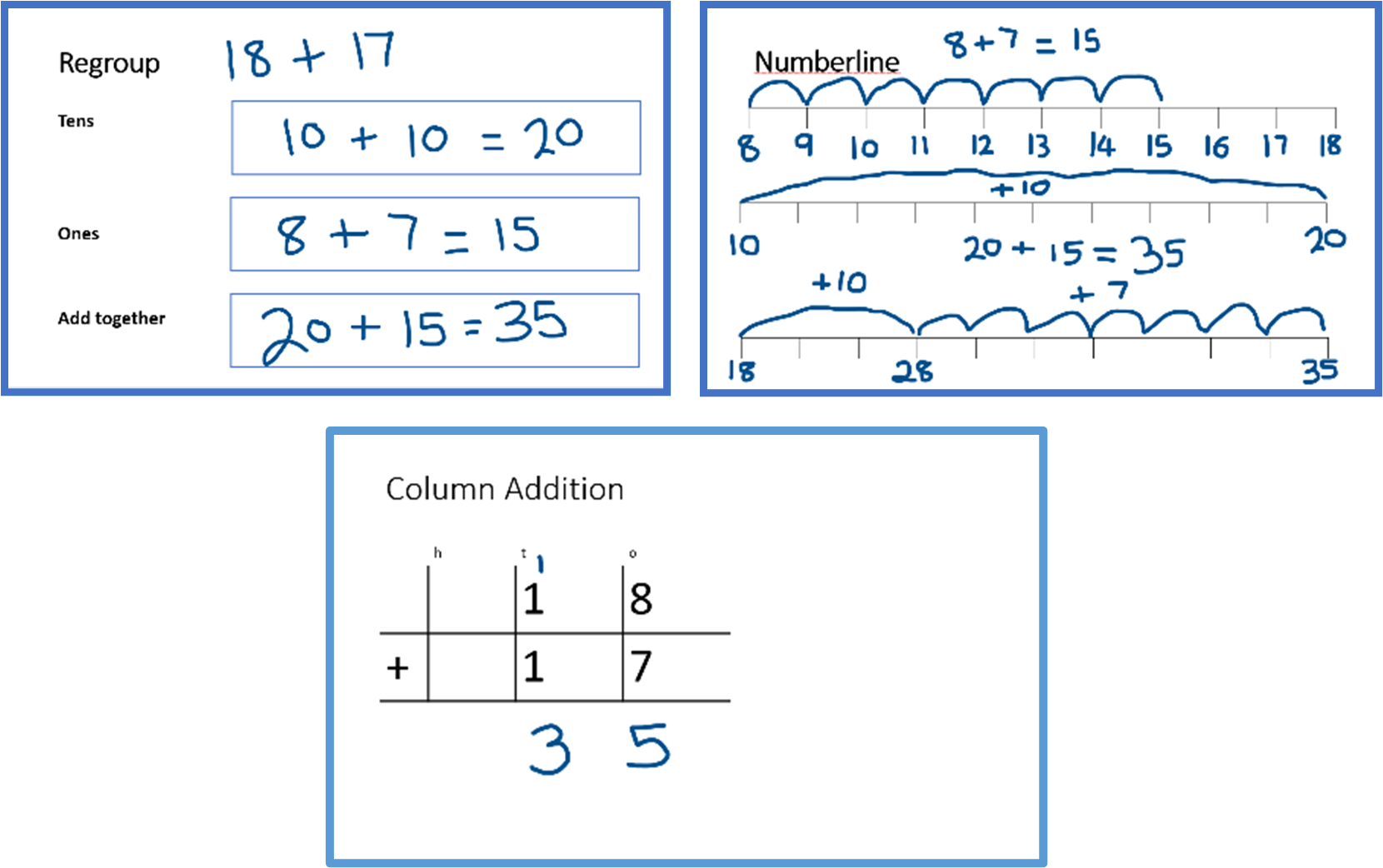
Remember a pupil doesn’t need to have a VI to find things visually confusing and sometimes recognising this can be the difference between them understanding or getting left behind in maths.
Guest blog authored by Siân Muggridge.


