
Background
Where did our number system come from?
Our base-10 number system was founded in India and is known as the Hindu Arabic Number System. Mathematicians used it in the Early Islamic civilisations, and it was fundamental to the mathematical discoveries in the House of Wisdom in Baghdad.
From there, mathematicians from Baghdad, such as Al Khawarizmi, introduced this system to Europe where it was quickly favoured over the much more complex Roman number system they had been using.
Did you know that algebra originated in the Early Islamic Civilisation, with Al Khwarizmi being coined the ‘father of algebra’?
Al Khawrizmi was fundamental to the introduction of algebra to Europe. By using algebra to support generalisations, he was able to formulate a range of equations and algorithms throughout his time working in the ‘House of Wisdom’.
Taking this into the maths classroom
Fundamentally, algebra is the way in which mathematicians can explain the patterns found in numbers and the way that they behave when manipulated. When you think about it in this way, children’s experience of algebra begins with noticing pattern in the early years and builds through primary to using simple formulae in year 6.
Below, I am going to explore a way of using algebra in the classroom in upper KS2.
First things first. If your school teaches children about the Early Islamic Civilisation in history, you could begin by making the link between what we do now, and how this has been influenced by the discoveries of mathematicians from this era, such as the previously mentioned Al Khawarizmi.
This could include:
- calculating exchange rates
- calculating taxes
- working with scale when designing new football stadiums or train stations.
Next, you could review simple formulae and model how you are able to swap unknown values for letters. This could be in the context of perimeter as a familiar concept from year 4, moving towards a different context such as finding a total cost.
Children could then be supported to ‘have a go’ at applying this to another area of maths - spotting patterns and reasoning using algebra.
Below are questions that you may like to try with your class.
Sammy noticed that when you square the number 4, the product is one more than when you multiply the numbers either side:
4 x 4 = 16
3 x 5 = 15
If you take a number and square it, is it always one more than when you multiply the numbers either side?
Why?
Build it, draw it, and see if you can use letters to show it.
Jeremiah noticed that the multiples of three follow a pattern:
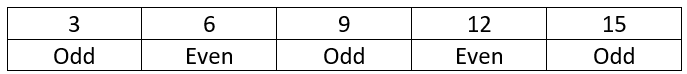
Jeremiah thinks this pattern is the same for the multiples of all odd numbers.
Do you agree?
Why?
Build it, draw it, and use letters to show it.
University of Cambridge: "Take Three Numbers" questions for your classroom in a printable sheet
Caution! Be prepared
Ensure you have had a go at the problem yourself first so that you can identify any possible barriers and scaffold the learning accordingly.
Speaking frames may be useful to support children in framing their thinking, or recording frames with familiar models to enable children to make connections.
To explore scaffolding further: Differentiation in maths - scaffolding or metaphorical escalators!
Support to develop reasoning and problem solving in your school
Find out how the HFL Education Primary Maths Team can work with you in school to develop reasoning and problem solving through the HFL Education Curriculum Impact Packages:
To keep up to date: Join our Primary Subject Leaders’ mailing list
To subscribe to our blogs: Get our blogs straight to your inbox
Originally published: 14/11/2023




